Question

In Figure 2, P (5, −3) and Q (3, y) are the points of trisection of the line segment joining A (7, −2) and B (1, −5). Then y equals

विकल्प

• A. 2

• B. 4

• C. −4

• D. `-5/2`

Answer 1

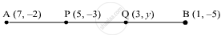
It is given that P and Q are the points of trisection of line segment AB.

∴ AP = PQ = QB

Now, AP = QB

`therefore sqrt((5-7)^2+(-3+2)^2)=sqrt((1-3)^2+(-5-y)^2)`

`rArrsqrt((-2)^2+(-1)^2)=sqrt((-2)+(5+y)^2)`

`rArrsqrt(4+1)=sqrt(4+25+y^2+10y)`

`rArrsqrt5=sqrt(y^2+10y+29)`

Squaring on both sides, we get

5 = y² + 10y + 29

∴ y² + 10y + 24 = 0

⇒ y² + 6y + 4y + 24 = 0

⇒ y(y + 6) + 4 (y + 6) = 0

⇒ (y + 4) (y + 6) = 0

⇒ y + 4 = 0 or y + 6 = 0

⇒ y = −4 or y = − 6

From the obtained values of y, − 4 matches with the option C.

Hence, the correct answer is C.