Advertisements
Advertisements
Question
In Figure 2, P (5, −3) and Q (3, y) are the points of trisection of the line segment joining A (7, −2) and B (1, −5). Then y equals

Options
A. 2
B. 4
C. −4
D. `-5/2`
Solution
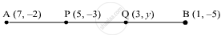
It is given that P and Q are the points of trisection of line segment AB.
∴ AP = PQ = QB
Now, AP = QB
`therefore sqrt((5-7)^2+(-3+2)^2)=sqrt((1-3)^2+(-5-y)^2)`
`rArrsqrt((-2)^2+(-1)^2)=sqrt((-2)+(5+y)^2)`
`rArrsqrt(4+1)=sqrt(4+25+y^2+10y)`
`rArrsqrt5=sqrt(y^2+10y+29)`
Squaring on both sides, we get
5 = y2 + 10y + 29
∴ y2 + 10y + 24 = 0
⇒ y2 + 6y + 4y + 24 = 0
⇒ y(y + 6) + 4 (y + 6) = 0
⇒ (y + 4) (y + 6) = 0
⇒ y + 4 = 0 or y + 6 = 0
⇒ y = −4 or y = − 6
From the obtained values of y, − 4 matches with the option C.
Hence, the correct answer is C.
APPEARS IN
RELATED QUESTIONS
The three vertices of a parallelogram taken in order are (–1, 0), (3, 1) and (2, 2) respectively. Find the coordinates of the fourth vertex.
Find the coordinates of the points of trisection of the line segment joining (4, -1) and (-2, -3).
Find the coordinates of a point A, where AB is the diameter of circle whose centre is (2, -3) and B is (1, 4).
If the points (-2, -1), (1, 0), (x, 3) and (1, y) form a parallelogram, find the values of x and y.
The line segment joining the points (3, -4) and (1, 2) is trisected at the points P and Q. If the coordinates of P and Q are (p, -2) and (5/3, q) respectively. Find the values of p and q.
In what ratio is the line joining A(0, 3) and B(4, –1) divided by the x-axis? Write the co-ordinates of the point where AB intersects the x-axis.
In what ratio is the line joining (2, -4) and (-3, 6) divided by the line y = O ?
The points A, B and C divides the line segment MN in four equal parts. The coordinates of Mand N are (-1, 10) and (7, -2) respectively. Find the coordinates of A, B and C.
If (2, 4) is the mid-point of the line segment joining (6, 3) and (a, 5), then the value of a is ______.
Find the co-ordinates of the points of trisection of the line segment joining the points (5, 3) and (4, 5).
