Advertisements
Advertisements
प्रश्न
A(6, -2), B(3, -2) and C(S, 6) are the three vertices of a parallelogram ABCD. Find the coordinates of the fourth vertex c.
उत्तर
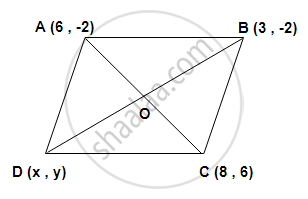
We know that in a parallelogram, diagonals bisect each other .
∴ midpoint of AC = midpoint of BD
14 = x + 3 , 4 = y - 2
x = 11 , y = 6
the coordinates of the fourth vertex Dare ( 11,6)
APPEARS IN
संबंधित प्रश्न
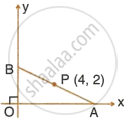
In the given figure, P(4, 2) is mid-point of line segment AB. Find the co-ordinates of A and B.
The points (2, –1), (–1, 4) and (–2, 2) are mid-points of the sides of a triangle. Find its vertices.
Complete the table below the graph with the help of the following graph.

| Sr. No. | First point | Second point | Co-ordinates of first point (x1 , y1) | Co-ordinates of second point (x2 , y2) | |
| 1 | C | E | (1, 0) | (3,4) | |
| 2 | A | B | (-1,-4) | (0,-2) | |
| 3 | B | D | (0,-2) | (2,2) |
Two vertices of a triangle are (1, 4) and (3, 1). If the centroid of the triangle is the origin, find the third vertex.
P , Q and R are collinear points such that PQ = QR . IF the coordinates of P , Q and R are (-5 , x) , (y , 7) , (1 , -3) respectively, find the values of x and y.
The centre ‘O’ of a circle has the coordinates (4, 5) and one point on the circumference is (8, 10). Find the coordinates of the other end of the diameter of the circle through this point.
The three vertices of a parallelogram taken in order are (-1, 0), (3, 1) and (2, 2) respectively. Find the coordinates of the fourth vertex.
The mid-point of the sides of a triangle are (2, 4), (−2, 3) and (5, 2). Find the coordinates of the vertices of the triangle
Find coordinates of midpoint of the segment joining points (0, 2) and (12, 14)
Point P is the centre of the circle and AB is a diameter. Find the coordinates of points B if coordinates of point A and P are (2, – 3) and (– 2, 0) respectively.
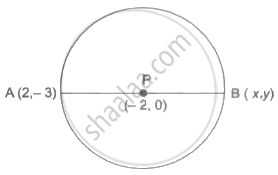
Given: A
The centre of the circle is the midpoint of the diameter.
∴ Mid point formula,
⇒
⇒ x =
⇒ x = – 6
and
⇒
⇒ y = 3
Hence coordinates of B is (– 6, 3).
