Advertisements
Advertisements
प्रश्न
A and B are two sets such that n(A – B) = 32 + x, n(B – A) = 5x and n(A ∩ B) = x. Illustrate the information by means of a Venn diagram. Given that n(A) = n(B). Calculate the value of x
उत्तर
n(A – B) = 32 + x, n(B – A) = 5x
n(A ∩ B) = x
From the Venn diagram:
Given n(A) = n(B)
32 + x + x = x + 5x
32 + 2x = 6x
32 = 6x – 2x
32 = 4x
x = `32/4` = 8
The value of x = 8
APPEARS IN
संबंधित प्रश्न
Given:
A = {Natural numbers less than 10}
B = {Letters of the word ‘PUPPET’}
C = {Squares of first four whole numbers}
D = {Odd numbers divisible by 2}.
Find: n(A)
Given:
A = {Natural numbers less than 10}
B = {Letters of the word ‘PUPPET’}
C = {Squares of first four whole numbers}
D = {Odd numbers divisible by 2}.
Find: n(C)
Given:
A = {Natural numbers less than 10}
B = {Letters of the word ‘PUPPET’}
C = {Squares of first four whole numbers}
D = {Odd numbers divisible by 2}.
Find: A ∩ C AND n(A ∩ C)
Given:
A = {Natural numbers less than 10}
B = {Letters of the word ‘PUPPET’}
C = {Squares of first four whole numbers}
D = {Odd numbers divisible by 2}.
Find: n(C ∩ D)
Given:
A = {Natural numbers less than 10}
B = {Letters of the word ‘PUPPET’}
C = {Squares of first four whole numbers}
D = {Odd numbers divisible by 2}.
Find: n(B ∪ C)
State true or false for the following. Correct the wrong statement.
If T ={a, l, a, h, b, d, h), then n(T) = 5
A survey of 1000 farmers found that 600 grew paddy, 350 grew ragi, 280 grew corn, 120 grew paddy and ragi, 100 grew ragi and corn, 80 grew paddy and corn. If each farmer grew atleast anyone of the above three, then find the number of farmers who grew all the three.
In the adjacent diagram, if n(U) = 125, y is two times of x and z is 10 more than x, then find the value of x, y and z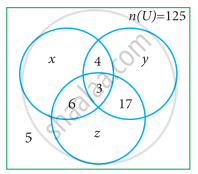
Each student in a class of 35 plays atleast one game among chess, carrom and table tennis. 22 play chess, 21 play carrom, 15 play table tennis, 10 play chess and table tennis, 8 play carrom and table tennis and 6 play all the three games. Find the number of students who play only carrom (Hint: Use Venn diagram)
If n(A ∪ B ∪ C) = 100, n(A) = 4x, n(B) = 6x, n(C) = 5x, n(A ∩ B) = 20, n(B ∩ C) = 15, n(A ∩ C) = 25 and n(A ∩ B ∩ C) = 10, then the value of x is
