Advertisements
Advertisements
प्रश्न
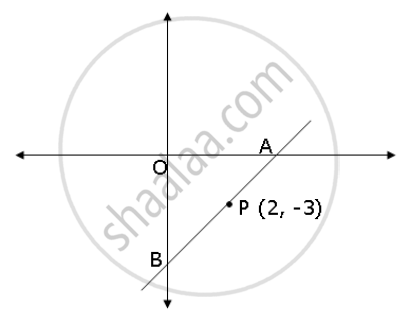
A and B are two points on the x-axis and y-axis respectively. P (2, −3) is the midpoint of AB. Find the:
(1) coordinates of A and B
(2) slope of line AB.
(3) an equation of line AB.

उत्तर
1) Let the coordinates be A(x, 0) and B(0, y).
Mid-point of A and B is given by `((x+0)/2 , (y + 0)/2) = (x/2, y/2)`
`=> (2, -3) = (x/2, y/2)`
=> `x/2 = 2` and `y/2 = -3`
=> x = 4 and y = 6
∴ A(4,0) and B(0,-6)
2) Slope of line AB, m = `(y_2 - y_1)/(x_2 - x_1) = (-6-0)/(0-4) = 3/2`
3) Equation of line AB, using A(4,0)
`y - 0 = 3/2 (x - 4) => 3x - 2y = 12`
APPEARS IN
संबंधित प्रश्न
A slope of a line is 3 and y-intercept is –4. Write the equation of a line
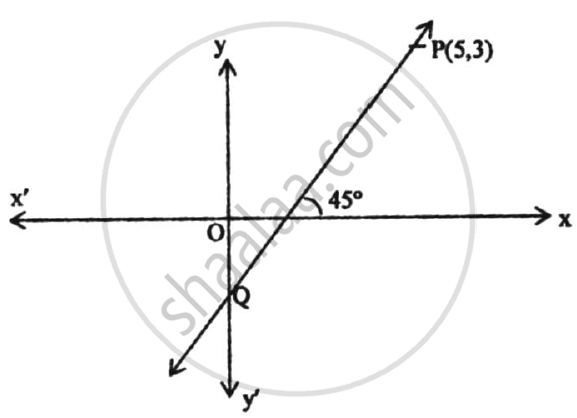
The line through P(5, 3) intersects y-axis at Q.
(1) Write the slope of the line.
(2) Write the equation of the line.
(3) Find the coordinates of Q.
The side AB of an equilateral triangle ABC is parallel to the x-axis. Find the slopes of all its sides.

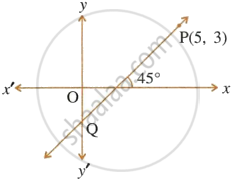
The line through P(5, 3) intersects y-axis at Q.
- Write the slope of the line.
- Write the equation of the line.
- Find the co-ordinates of Q.
The lines represented by 4x + 3y = 9 and px – 6y + 3 = 0 are parallel. Find the value of p.
Determine whether the given point is collinear.
\[P\left( 1, 2 \right), Q\left( 2, \frac{8}{5} \right), R\left( 3, \frac{6}{5} \right)\]
Find the slope and the y-intercept of the following line 2x + 3y = 12
Find slope of a line passing through the points A(3, 1) and B(5, 3).
With out Pythagoras theorem, show that A(4, 4), B(3, 5) and C(-1, -1) are the vertices of a right angled.
Show that the points A(- 2, 5), B(2, – 3) and C(0, 1) are collinear.
