Advertisements
Advertisements
प्रश्न
A ball is thrown vertically upwards. It goes to a height 20 m and then returns to the ground. Taking acceleration due to gravity g to be 10 ms-2 , find :
the final velocity of the ball on reaching the ground .
उत्तर
Given, maximum height reached, s = 20 m
Acceleration due to gravity, g = 10 m/s2
Let v' be the final velocity of the ball on reaching the ground.
Considering the motion from the highest point to ground,
Velocity at highest point = 0 = Initial velocity for downward journey of the ball.
Distance travelled, s = 20m
Using the third equation of motion,
v2 - u2 = 2gs
or, v2 - 0 = 2 (10) (20) m/s
or, v2 = 400 m/s
or, v = 20 m/s
APPEARS IN
संबंधित प्रश्न
Choose the correct alternative:
Acceleration due to gravity increases/decreases with increasing depth. (assume the earth to be a sphere of uniform density).
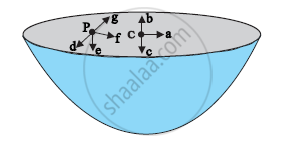
The gravitational intensity at the centre of a hemispherical shell of uniform mass density has the direction indicated by the arrow (see Fig 8.12) (i) a, (ii) b, (iii) c, (iv) 0.
Can you think of two particles which do not exert gravitational force on each other?
A body is suspended from a spring balance kept in a satellite. The reading of the balance is W1 when the satellite goes in an orbit of radius R and is W2 when it goes in an orbit of radius 2 −R.
The mass of moon is about 0.012 times that of earth and its diameter is about 0.25 times that of earth. The value of G on the moon will be:
A ball is thrown vertically upwards. It goes to a height 20 m and then returns to the ground. Taking acceleration due to gravity g to be 10 ms-2 , find :
the total time of journey of the ball .
Where will you weigh more: at the centre of the earth or at the surface of the earth?
What is meant by the equation :
`g= Gxxm/r^2`
where the symbols have their usual meanings.
State Newton's law of gravitation. What is the difference between:
Gravity and gravitation
Molecules in air in the atmosphere are attracted by gravitational force of the earth. Explain why all of them do not fall into the earth just like an apple falling from a tree.
