Advertisements
Advertisements
प्रश्न
A circular park of radius 40 m is situated in a colony. Three boys Ankur, Amit and Anand are sitting at equal distance on its boundary each having a toy telephone in his hands to talk
to each other.
उत्तर
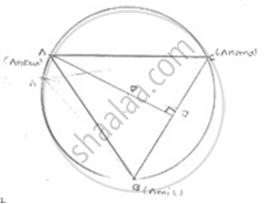
Given hat AB = BC = CA
So, ABC is an equilateral triangle
OA (radius) = 40m.
Medians of equilaterals triangles pass through the circum center (0) of the equilaterals
triangles ABC
We also know that median intersect each other at the 2 :1As AD is the median of equilaterals triangle ABC, we can write:
`(OA)/(OD)-2/7`
`⇒(40m)/(OD)-2/7`
`∴AD=OA+OD=(40+20)m`
`=60m`
In ΔADC
By using Pythagoras theorem
`AC^2=AD^2+DC^2`
`AC^2=AD^2+DC^2`
`AC^2=(60)^2+((AC)/2)^2`
`AC^2=3600+(AC^2)/4`
`⇒3/2AC^2=3600`\
`⇒AC^2=4800`
`⇒AC^2=40sqrt3m`
So, length of string of each phone will be `40sqrt3m`
APPEARS IN
संबंधित प्रश्न
Draw different pairs of circles. How many points does each pair have in common? What is the maximum number of common points?
If two circles intersect at two points, prove that their centres lie on the perpendicular bisector of the common chord.
Fill in the blank:
All points lying inside/outside a circle are called .................. points/ .....................points.
Give a method to find the centre of a given circle.
Prove that any three points on a circle cannot be collinear.
Choose the correct alternative:
If the points, A, B, C are non-collinear points, then how many circles can be drawn which passes through points A, B, and C?
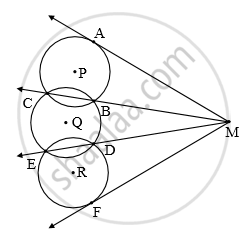
In the above figure, the circles with P, Q, and R intersect at points B, C, D, and E as shown. Lines CB and ED intersect in point M. Lines are drawn from point M to touch the circles at points A and F. Prove that MA = MF.
How many circles can be drawn passing through a point?
Through three collinear points a circle can be drawn.
Find the value of y, if the points A(3, 4), B(6, y) and C(7, 8) are collinear.
