Advertisements
Advertisements
प्रश्न
A conducting loop of area 5.0 cm2 is placed in a magnetic field which varies sinusoidally with time as B = B0 sin ωt where B0 = 0.20 T and ω = 300 s−1. The normal to the coil makes an angle of 60° with the field. Find (a) the maximum emf induced in the coil, (b) the emf induced at τ = (π/900)s and (c) the emf induced at t = (π/600) s.
उत्तर
Given:-
Area of the coil, A = 5 cm2 = 5 × 10−4 m2
The magnetic field at time t is given by
B = B0 sin ωt = 0.2 sin (300t)
Angle of the normal of the coil with the magnetic field, θ = 60°
(a) The emf induced in the coil is given by
\[e = \frac{- d\theta}{dt} = \frac{d}{dt}(BA \cos \theta)\]
\[ = \frac{d}{dt}\left[ \left( B_0 \sin \omega t \right) \times 5 \times {10}^{- 4} \times 1/2 \right]\]
\[ = B_0 \times \frac{5}{2} \times {10}^{- 4} \frac{d}{dt}(\sin \omega t)\]
\[ = \frac{B_0 5}{2} {10}^{- 4} \omega\left( \cos \omega t \right)\]
\[ = \frac{0 . 2 \times 5}{2} \times 300 \times {10}^{- 4} \times \cos \omega t\]
\[ = 15 \times {10}^{- 3} cost \omega t\]
The induced emf becomes maximum when cos ωt becomes maximum, that is, 1.
Thus, the maximum value of the induced emf is given by
\[e_{max} = 15 \times {10}^{- 3} = 0 . 015 V\]
(b) The induced emf at t = \[\left( \frac{\pi}{900} \right) s\] is given by
e = 15 × 10−3 × cos ωt
= 15 × 10−3 × cos\[\left( 300\times\frac{\pi}{900} \right)\]
= 15 × 10−3 × `1/2`
\[= \frac{0 . 015}{2} = 0 . 0075 = 7 . 5 \times {10}^{- 3} V\]
(c) The induced emf at t = \[\frac{\pi}{600} s\] is given by
e = 15 × 10−3 × cos \[\left( 300 \times \frac{\pi}{600} \right)\]
= 15 × 10−3 × 0 = 0 V
APPEARS IN
संबंधित प्रश्न
The current flowing through an inductor of self-inductance L is continuously increasing. Plot a graph showing the variation of
Induced emf versus dI/dt
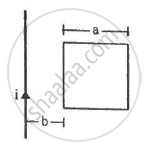
(a) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side an as shown in the figure.
(b) Now assume that the straight wire carries a current of 50 A and the loop is moved to the right with a constant velocity, v = 10 m/s.
Calculate the induced emf in the loop at the instant when x = 0.2 m.
Take a = 0.1 m and assume that the loop has a large resistance.

A circular coil of radius 10 cm, 500 turns and resistance 200 Ω is placed with its plane perpendicular to the horizontal component of the Earth's magnetic field. It is rotated about its vertical diameter through 180° in 0.25 s. Estimate the magnitude of the emf and current induced in the coil. (Horizontal component of the Earth's magnetic field at the place is 3.0 ✕ 10−5 T).
A metallic rod of length ‘l’ is rotated with a frequency v with one end hinged at the centre and the other end at the circumference of a circular metallic ring of radius r, about an axis passing through the centre and perpendicular to the plane of the ring. A constant uniform magnetic field B parallel to the axis is present everywhere. Using Lorentz force, explain how emf is induced between the centre and the metallic ring and hence obtained the expression for it.
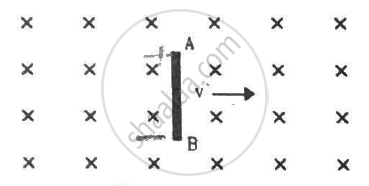
A rod AB moves with a uniform velocity v in a uniform magnetic field as shown in figure.
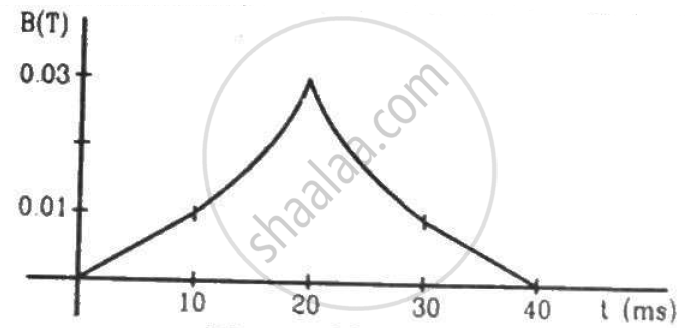
(a) The magnetic field in a region varies as shown in figure. Calculate the average induced emf in a conducting loop of area 2.0 × 10−3 m2 placed perpendicular to the field in each of the 10 ms intervals shown. (b) In which intervals is the emf not constant? Neglect the behaviour near the ends of 10 ms intervals.
Suppose the resistance of the coil in the previous problem is 25Ω. Assume that the coil moves with uniform velocity during its removal and restoration. Find the thermal energy developed in the coil during (a) its removal, (b) its restoration and (c) its motion.
A conducting loop of face-area A and resistance R is placed perpendicular to a magnetic field B. The loop is withdrawn completely from the field. Find the charge which flows through any cross-section of the wire in the process. Note that it is independent of the shape of the loop as well as the way it is withdrawn.
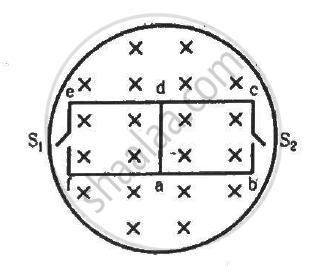
The magnetic field in the cylindrical region shown in figure increases at a constant rate of 20.0 mT/s. Each side of the square loop abcd and defa has a length of 1.00 cm and a resistance of 4.00 Ω. Find the current (magnitude and sense) in the wire ad if (a) the switch S1 is closed but S2 is open, (b) S1 is open but S2 is closed, (c) both S1 and S2 are open and (d) both S1 and S2 are closed.
A wire of length 10 cm translates in a direction making an angle of 60° with its length. The plane of motion is perpendicular to a uniform magnetic field of 1.0 T that exists in the space. Find the emf induced between the ends of the rod if the speed of translation is 20 cm s−1.
A rod of length l rotates with a uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the rod is ___________ .
The current generator Ig' shown in figure, sends a constant current i through the circuit. The wire cd is fixed and ab is made to slide on the smooth, thick rails with a constant velocity v towards right. Each of these wires has resistance r. Find the current through the wire cd.

A bicycle is resting on its stand in the east-west direction and the rear wheel is rotated at an angular speed of 100 revolutions per minute. If the length of each spoke is 30.0 cm and the horizontal component of the earth's magnetic field is 2.0 × 10−5 T, find the emf induced between the axis and the outer end of a spoke. Neglect centripetal force acting on the free electrons of the spoke.
Figure shows a square frame of wire having a total resistance r placed coplanarly with a long, straight wire. The wire carries a current i given by i = i0 sin ωt. Find (a) the flux of the magnetic field through the square frame, (b) the emf induced in the frame and (c) the heat developed in the frame in the time interval 0 to \[\frac{20\pi}{\omega}.\]
A wire of mass m and length l can slide freely on a pair of smooth, vertical rails (figure). A magnetic field B exists in the region in the direction perpendicular to the plane of the rails. The rails are connected at the top end by a capacitor of capacitance C. Find the acceleration of the wire neglecting any electric resistance.

A small flat search coil of area 5cm2 with 140 closely wound turns is placed between the poles of a powerful magnet producing magnetic field 0.09T and then quickly removed out of the field region. Calculate:
(a) Change of magnetic flux through the coil, and
(b) emf induced in the coil.
Plot a graph showing variation of induced e.m.f. with the rate of change of current flowing through a coil.
Two identical coaxial coils P and Q carrying equal amount of current in the same direction are brought nearer. The current in ______.
When the rate of change oic current is unity, the induced emf is equal to ______.
