Advertisements
Advertisements
प्रश्न
A cubic vessel (with faces horizontal + vertical) contains an ideal gas at NTP. The vessel is being carried by a rocket which is moving at a speed of 500 ms–1 in vertical direction. The pressure of the gas inside the vessel as observed by us on the ground ______.
विकल्प
remains the same because 500 ms−1 is very much smaller than vrms of the gas.
remains the same because motion of the vessel as a whole does not affect the relative motion of the gas molecules and the walls.
will increase by a factor equal to `(v_(rms)^2 + (500)^2)/v_(rms)^2` where vrms was the original mean square velocity of the gas.
will be different on the top wall and bottom wall of the vessel.
उत्तर
A cubic vessel (with faces horizontal + vertical) contains an ideal gas at NTP. The vessel is being carried by a rocket which is moving at a speed of 500 ms–1 in vertical direction. The pressure of the gas inside the vessel as observed by us on the ground remains the same because motion of the vessel as a whole does not affect the relative motion of the gas molecules and the walls.
Explanation:
According to the ideal gas law,
P = nRT/V, here temperature of the vessel remains unchanged hence, the pressure remains the same from that point of view.
Now, let us discuss the phenomenon inside the vessel. The gas molecules keep on colliding among themselves as well as with the walls of containing vessel. These collisions are perfectly elastic.
The number of collisions per unit volume in a gas remains constant. So, the pressure of the gas inside the vessel remains the same because the motion of the vessel as a whole does not affect the relative motion of the gas molecules with respect to the walls.
APPEARS IN
संबंधित प्रश्न
Which of the following quantities is zero on an average for the molecules of an ideal gas in equilibrium?
Keeping the number of moles, volume and temperature the same, which of the following are the same for all ideal gases?
The average momentum of a molecule in a sample of an ideal gas depends on
Let Q and W denote the amount of heat given to an ideal gas and the work done by it in an adiabatic process.
(a) Q = 0
(b) W = 0
(c) Q = W
(d) Q ≠ W
A vessel containing one mole of a monatomic ideal gas (molecular weight = 20 g mol−1) is moving on a floor at a speed of 50 m s−1. The vessel is stopped suddenly. Assuming that the mechanical energy lost has gone into the internal energy of the gas, find the rise in its temperature.
The ratio of the molar heat capacities of an ideal gas is Cp/Cv = 7/6. Calculate the change in internal energy of 1.0 mole of the gas when its temperature is raised by 50 K (a) keeping the pressure constant (b) keeping the volume constant and (c) adiaba
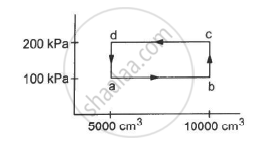
Half mole of an ideal gas (γ = 5/3) is taken through the cycle abcda, as shown in the figure. Take `"R" = 25/3"J""K"^-1 "mol"^-1 `. (a) Find the temperature of the gas in the states a, b, c and d. (b) Find the amount of heat supplied in the processes ab and bc. (c) Find the amount of heat liberated in the processes cd and da.
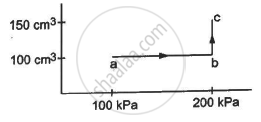
An ideal gas (γ = 1.67) is taken through the process abc shown in the figure. The temperature at point a is 300 K. Calculate (a) the temperatures at b and c (b) the work done in the process (c) the amount of heat supplied in the path ab and in the path bcand (d) the change in the internal energy of the gas in the process.
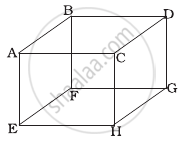
1 mole of an ideal gas is contained in a cubical volume V, ABCDEFGH at 300 K (Figure). One face of the cube (EFGH) is made up of a material which totally absorbs any gas molecule incident on it. At any given time ______.
The container shown in figure has two chambers, separated by a partition, of volumes V1 = 2.0 litre and V2 = 3.0 litre. The chambers contain µ1 = 4.0 and µ2 = 5.0 moles of a gas at pressures p1 = 1.00 atm and p2 = 2.00 atm. Calculate the pressure after the partition is removed and the mixture attains equilibrium.
| V1 | V2 |
| µ1, p1 | µ2 |
| p2 |
