Advertisements
Advertisements
प्रश्न
The container shown in figure has two chambers, separated by a partition, of volumes V1 = 2.0 litre and V2 = 3.0 litre. The chambers contain µ1 = 4.0 and µ2 = 5.0 moles of a gas at pressures p1 = 1.00 atm and p2 = 2.00 atm. Calculate the pressure after the partition is removed and the mixture attains equilibrium.
| V1 | V2 |
| µ1, p1 | µ2 |
| p2 |
उत्तर
Consider the diagram,
| p1V1 | p2V2 |
| µ1 | µ2 |
Given, V1 = 2.0 L, V2 = 3.0 L
µ1 = 4.0 mol, µ2 = 5.0 mol
p1 = 1.00 atm, p2 = 2.00 atm
For chamber 1, p1, V1 = µ1RT1
For chamber 2, p2, V2 = µ2RT2
When the partition is removed the gases get mixed without any loss of energy. The mixture Now attains a common equilibrium pressure and the total volume of the system is the sum of the volume of individual chambers V1 and V2.
So, µ = µ1 + µ2, V = V1 + V2
From the kinetic theory of gases,
For `l` mole `pV = 2/3 E` ......`[(E = "Translational"),("Kinetic energy")]`
For `µ_1` moles, `p_1V_1 = 2/3 µ_1E_1`
For `µ_2` moles, `p_2V_2 = 2/3 µ_2E_2`
The total energy is `(µ_1E_1 + µ_2E_2) = 3/2 (p_1V_1 + p_2V_2)`
From the above relation, `pV = 2/3 E_"total" = 2/3 µE_"per mole"`
`p(V_1 + V_2) = 2/3 xx 3/2 (p_1V_1 + p_2V_2)`
`p = (p_1V_1 + p_2V_2)/(V_1 + V_2)`
= `((1.00 + 2.0 + 2.00 xx 3.0)/(2.0 + 3.0))` atm
= `8.0/5.0`
= 1.60 atm
APPEARS IN
संबंधित प्रश्न
Which of the following quantities is zero on an average for the molecules of an ideal gas in equilibrium?
A sample of 0.177 g of an ideal gas occupies 1000 cm3 at STP. Calculate the rms speed of the gas molecules.
A vessel containing one mole of a monatomic ideal gas (molecular weight = 20 g mol−1) is moving on a floor at a speed of 50 m s−1. The vessel is stopped suddenly. Assuming that the mechanical energy lost has gone into the internal energy of the gas, find the rise in its temperature.
Two ideal gases have the same value of Cp / Cv = γ. What will be the value of this ratio for a mixture of the two gases in the ratio 1 : 2?
1 litre of an ideal gas (γ = 1.5) at 300 K is suddenly compressed to half its original volume. (a) Find the ratio of the final pressure to the initial pressure. (b) If the original pressure is 100 kPa, find the work done by the gas in the process. (c) What is the change in internal energy? (d) What is the final temperature? (e) The gas is now cooled to 300 K keeping its pressure constant. Calculate the work done during the process. (f) The gas is now expanded isothermally to achieve its original volume of 1 litre. Calculate the work done by the gas. (g) Calculate the total work done in the cycle.
An ideal gas of density 1.7 × 10−3 g cm−3 at a pressure of 1.5 × 105 Pa is filled in a Kundt's tube. When the gas is resonated at a frequency of 3.0 kHz, nodes are formed at a separation of 6.0 cm. Calculate the molar heat capacities Cp and Cv of the gas.
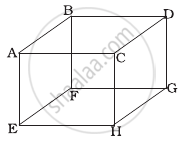
1 mole of an ideal gas is contained in a cubical volume V, ABCDEFGH at 300 K (Figure). One face of the cube (EFGH) is made up of a material which totally absorbs any gas molecule incident on it. At any given time ______.
Diatomic molecules like hydrogen have energies due to both translational as well as rotational motion. From the equation in kinetic theory `pV = 2/3` E, E is ______.
- the total energy per unit volume.
- only the translational part of energy because rotational energy is very small compared to the translational energy.
- only the translational part of the energy because during collisions with the wall pressure relates to change in linear momentum.
- the translational part of the energy because rotational energies of molecules can be of either sign and its average over all the molecules is zero.
When an ideal gas is compressed adiabatically, its temperature rises: the molecules on the average have more kinetic energy than before. The kinetic energy increases ______.
- because of collisions with moving parts of the wall only.
- because of collisions with the entire wall.
- because the molecules gets accelerated in their motion inside the volume.
- because of redistribution of energy amongst the molecules.
We have 0.5 g of hydrogen gas in a cubic chamber of size 3 cm kept at NTP. The gas in the chamber is compressed keeping the temperature constant till a final pressure of 100 atm. Is one justified in assuming the ideal gas law, in the final state?
(Hydrogen molecules can be consider as spheres of radius 1 Å).
