Advertisements
Advertisements
प्रश्न
A vessel containing one mole of a monatomic ideal gas (molecular weight = 20 g mol−1) is moving on a floor at a speed of 50 m s−1. The vessel is stopped suddenly. Assuming that the mechanical energy lost has gone into the internal energy of the gas, find the rise in its temperature.
उत्तर
Number of moles of the ideal gas, n = 1 mole
Molecular weight of the gas, W = 20 g/mole
Mass of the gas, m =20 g
Velocity of the vessel, V = 50 m/s
Decrease in K.E. of the vessel = Internal energy gained by the gas
`"K""E" = 1/2 "m"("u"^2 -"v"^2)`
`"K""E" = 1/2 xx 20 xx 10^-3 xx (0-50 xx 50)`
KE = -25 J = gain in internal energy of the gas change in internal energy of a gas `d/2n R(triangle"T")`
where d is the degree of freedom of the gas F or a monoatomic gas , d=3.
So, 25 = ` 3/2 n R (triangle T)`
`=> 25 = 1 xx 3/2 xx 8.31 xx triangle T`
`=> triangle T = 50 /(3 xx 8.3) = 2 "K"`
APPEARS IN
संबंधित प्रश्न
The energy of a given sample of an ideal gas depends only on its
The average momentum of a molecule in a sample of an ideal gas depends on
Calculate the volume of 1 mole of an ideal gas at STP.
Find the number of molecules in 1 cm3 of an ideal gas at 0°C and at a pressure of 10−5mm of mercury.
Use R = 8.31 J K-1 mol-1
Let Q and W denote the amount of heat given to an ideal gas and the work done by it in an isothermal process.
The ratio of the molar heat capacities of an ideal gas is Cp/Cv = 7/6. Calculate the change in internal energy of 1.0 mole of the gas when its temperature is raised by 50 K (a) keeping the pressure constant (b) keeping the volume constant and (c) adiaba
An ideal gas is taken through a process in which the pressure and the volume are changed according to the equation p = kV. Show that the molar heat capacity of the gas for the process is given by `"C" ="C"_"v" +"R"/2.`
Two ideal gases have the same value of Cp / Cv = γ. What will be the value of this ratio for a mixture of the two gases in the ratio 1 : 2?
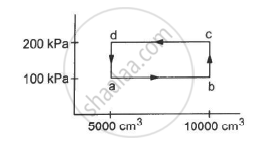
Half mole of an ideal gas (γ = 5/3) is taken through the cycle abcda, as shown in the figure. Take `"R" = 25/3"J""K"^-1 "mol"^-1 `. (a) Find the temperature of the gas in the states a, b, c and d. (b) Find the amount of heat supplied in the processes ab and bc. (c) Find the amount of heat liberated in the processes cd and da.
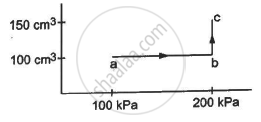
An ideal gas (γ = 1.67) is taken through the process abc shown in the figure. The temperature at point a is 300 K. Calculate (a) the temperatures at b and c (b) the work done in the process (c) the amount of heat supplied in the path ab and in the path bcand (d) the change in the internal energy of the gas in the process.
An ideal gas at pressure 2.5 × 105 Pa and temperature 300 K occupies 100 cc. It is adiabatically compressed to half its original volume. Calculate (a) the final pressure (b) the final temperature and (c) the work done by the gas in the process. Take γ = 1.5
Two samples A and B, of the same gas have equal volumes and pressures. The gas in sample A is expanded isothermally to double its volume and the gas in B is expanded adiabatically to double its volume. If the work done by the gas is the same for the two cases, show that γ satisfies the equation 1 − 21−γ = (γ − 1) ln2.
1 litre of an ideal gas (γ = 1.5) at 300 K is suddenly compressed to half its original volume. (a) Find the ratio of the final pressure to the initial pressure. (b) If the original pressure is 100 kPa, find the work done by the gas in the process. (c) What is the change in internal energy? (d) What is the final temperature? (e) The gas is now cooled to 300 K keeping its pressure constant. Calculate the work done during the process. (f) The gas is now expanded isothermally to achieve its original volume of 1 litre. Calculate the work done by the gas. (g) Calculate the total work done in the cycle.
Figure shows a cylindrical tube with adiabatic walls and fitted with an adiabatic separator. The separator can be slid into the tube by an external mechanism. An ideal gas (γ = 1.5) is injected in the two sides at equal pressures and temperatures. The separator remains in equilibrium at the middle. It is now slid to a position where it divides the tube in the ratio 1 : 3. Find the ratio of the temperatures in the two parts of the vessel.

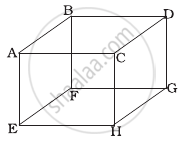
1 mole of an ideal gas is contained in a cubical volume V, ABCDEFGH at 300 K (Figure). One face of the cube (EFGH) is made up of a material which totally absorbs any gas molecule incident on it. At any given time ______.
ABCDEFGH is a hollow cube made of an insulator (Figure). Face ABCD has positive charge on it. Inside the cube, we have ionized hydrogen. The usual kinetic theory expression for pressure ______.
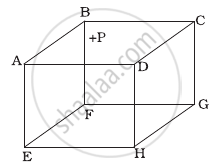
- will be valid.
- will not be valid since the ions would experience forces other than due to collisions with the walls.
- will not be valid since collisions with walls would not be elastic.
- will not be valid because isotropy is lost.
