Advertisements
Advertisements
प्रश्न
The container shown in figure has two chambers, separated by a partition, of volumes V1 = 2.0 litre and V2 = 3.0 litre. The chambers contain µ1 = 4.0 and µ2 = 5.0 moles of a gas at pressures p1 = 1.00 atm and p2 = 2.00 atm. Calculate the pressure after the partition is removed and the mixture attains equilibrium.
| V1 | V2 |
| µ1, p1 | µ2 |
| p2 |
उत्तर
Consider the diagram,
| p1V1 | p2V2 |
| µ1 | µ2 |
Given, V1 = 2.0 L, V2 = 3.0 L
µ1 = 4.0 mol, µ2 = 5.0 mol
p1 = 1.00 atm, p2 = 2.00 atm
For chamber 1, p1, V1 = µ1RT1
For chamber 2, p2, V2 = µ2RT2
When the partition is removed the gases get mixed without any loss of energy. The mixture Now attains a common equilibrium pressure and the total volume of the system is the sum of the volume of individual chambers V1 and V2.
So, µ = µ1 + µ2, V = V1 + V2
From the kinetic theory of gases,
For `l` mole `pV = 2/3 E` ......`[(E = "Translational"),("Kinetic energy")]`
For `µ_1` moles, `p_1V_1 = 2/3 µ_1E_1`
For `µ_2` moles, `p_2V_2 = 2/3 µ_2E_2`
The total energy is `(µ_1E_1 + µ_2E_2) = 3/2 (p_1V_1 + p_2V_2)`
From the above relation, `pV = 2/3 E_"total" = 2/3 µE_"per mole"`
`p(V_1 + V_2) = 2/3 xx 3/2 (p_1V_1 + p_2V_2)`
`p = (p_1V_1 + p_2V_2)/(V_1 + V_2)`
= `((1.00 + 2.0 + 2.00 xx 3.0)/(2.0 + 3.0))` atm
= `8.0/5.0`
= 1.60 atm
APPEARS IN
संबंधित प्रश्न
Calculate the volume of 1 mole of an ideal gas at STP.
A rigid container of negligible heat capacity contains one mole of an ideal gas. The temperature of the gas increases by 1° C if 3.0 cal of heat is added to it. The gas may be
(a) helium
(b) argon
(c) oxygen
(d) carbon dioxide
An amount Q of heat is added to a monatomic ideal gas in a process in which the gas performs a work Q/2 on its surrounding. Find the molar heat capacity for the process
An ideal gas is taken through a process in which the pressure and the volume are changed according to the equation p = kV. Show that the molar heat capacity of the gas for the process is given by `"C" ="C"_"v" +"R"/2.`
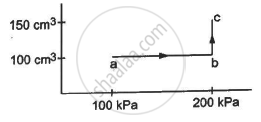
An ideal gas (γ = 1.67) is taken through the process abc shown in the figure. The temperature at point a is 300 K. Calculate (a) the temperatures at b and c (b) the work done in the process (c) the amount of heat supplied in the path ab and in the path bcand (d) the change in the internal energy of the gas in the process.
An ideal gas at pressure 2.5 × 105 Pa and temperature 300 K occupies 100 cc. It is adiabatically compressed to half its original volume. Calculate (a) the final pressure (b) the final temperature and (c) the work done by the gas in the process. Take γ = 1.5
Consider a given sample of an ideal gas (Cp/Cv = γ) having initial pressure p0 and volume V0. (a) The gas is isothermally taken to a pressure p0/2 and from there, adiabatically to a pressure p0/4. Find the final volume. (b) The gas is brought back to its initial state. It is adiabatically taken to a pressure p0/2 and from there, isothermally to a pressure p0/4. Find the final volume.
Two vessels A and B of equal volume V0 are connected by a narrow tube that can be closed by a valve. The vessels are fitted with pistons that can be moved to change the volumes. Initially, the valve is open and the vessels contain an ideal gas (Cp/Cv = γ) at atmospheric pressure p0 and atmospheric temperature T0. The walls of vessel A are diathermic and those of B are adiabatic. The valve is now closed and the pistons are slowly pulled out to increase the volumes of the vessels to double the original value. (a) Find the temperatures and pressures in the two vessels. (b) The valve is now opened for sufficient time so that the gases acquire a common temperature and pressure. Find the new values of the temperature and pressure.
The figure shows an adiabatic cylindrical tube of volume V0 divided in two parts by a frictionless adiabatic separator. Initially, the separator is kept in the middle, an ideal gas at pressure p1 and temperature T1 is injected into the left part and another ideal gas at pressure p2 and temperature T2 is injected into the right part. Cp/Cv = γ is the same for both the gases. The separator is slid slowly and is released at a position where it can stay in equilibrium. Find (a) the volumes of the two parts (b) the heat given to the gas in the left part and (c) the final common pressure of the gases.

An ideal gas of density 1.7 × 10−3 g cm−3 at a pressure of 1.5 × 105 Pa is filled in a Kundt's tube. When the gas is resonated at a frequency of 3.0 kHz, nodes are formed at a separation of 6.0 cm. Calculate the molar heat capacities Cp and Cv of the gas.
