Advertisements
Advertisements
प्रश्न
A four-digit number abcd is divisible by 11, if d + b = ______ or _____.
उत्तर
A four-digit number abcd is divisible by 11, if d + b = a + c or 12(a + c).
Explanation:
We know that, a number is divisible by 11, if the difference between the sum of digits at odd places and the sum of its digits at even places is either 0 or a multiple of 11.
Hence, abcd is divisible by 11, if (d + b) – (a + c) = 0, 11, 22, 33, ...
⇒ d + b = a + c or d + b = 12(a + c)
APPEARS IN
संबंधित प्रश्न
Which of the following statement is true?
If a number is divisible by 8, it must be divisible by 4.
Which of the following statement is true?
If a number exactly divides the sum of two numbers, it must exactly divide the numbers separately.
Use the same rule to fill the hexagons below.
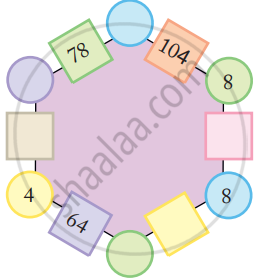
Now you also make your own magic hexagons.
Now, look at this —
![]()
Check if it is true or not.
Number 7N + 1 will leave remainder 1 when divided by 7.
If AB × 4 = 192, then A + B = 7.
If N ÷ 5 leaves remainder 3 and N ÷ 2 leaves remainder 0, then N ÷ 10 leaves remainder 4.
Find the least value that must be given to number a so that the number 91876a2 is divisible by 8.
If 148101B095 is divisible by 33, find the value of B.
Fill in the blank space in the same way.