Question

A line l is parallel to line m and a transversal p intersects them at X, Y respectively. Bisectors of interior angles at X and Y interesct at P and Q. Is PXQY a rectangle? Given reason.

Answer 1

Given, l || m

Now, ∠DXY = ∠XYA   ...[Alternate interior angles]

⇒ `(∠DXY)/2 = (∠XYA)/2` ...[Dividing both the sides by 2]

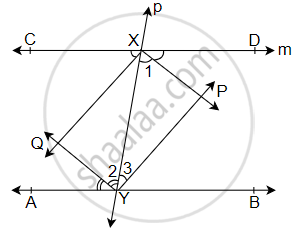
Now, ∠1 = ∠2   ...[Alternate angle are equal]

XP and YQ are bisectors.

So, XP || QY  ...(i)

Similarly, XQ || PY  ...(ii)

Now, from equation (i) and (ii), we get

In parallelogram PXQY,

∠DXY + ∠XYB = 180°   ...(iii) [Interior angles on the same side of transversal are supplementary]

Now, dividing both the sides by 2, get

`(∠DXY)/2 + (∠XYB)/2 = 180^circ/2`  

So, ∠1 + ∠3 = 90°   [Dividing both the sides by 2] ...(iv)

In triangle XYP,

∠1 + ∠3 + ∠P = 180°

90° + ∠P = 180°   ...[From equation (iv)]

∠P = 180° – 90°

∠P = 90°  ...(v)

From equations (iii) and (v),

PXQY is a rectangle.