Advertisements
Advertisements
प्रश्न
The sides of a rectangle are in the ratio 2 : 3, and its perimeter is 20 cm. Draw the rectangle.
उत्तर
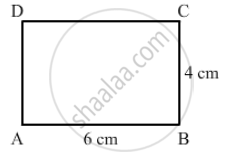
\[\text{ Let the side be x cm and y cm } . \]
\[\text{ So, we have }: \]
\[ 2\left( x + y \right) = 20 \]
\[\text{ Sides are in the ratio 2: 3 } . \]
\[ \therefore y = \frac{3x}{2}\]
\[\text{ Putting the value of y }: \]
\[2\left( x + \frac{3x}{2} \right) = 20 \]
\[\frac{2x + 3x}{2} = 10\]
\[5x = 20\]
\[x = 4\]
\[ \therefore y = \frac{3 \times 4}{2} = 6\]
\[\text{ Thus, sides of the rectangle will be 4 cm and 6 cm } . \]
\[\text{ ABCD is the rectangle having sides 4 cm and 6 cm } .\]
APPEARS IN
संबंधित प्रश्न
Diagonals of a parallelogram ABCD intersect at O. AL and CM are drawn perpendiculars to BD such that L and M lie on BD. Is AL = CM? Why or why not?
Which of the following statement is true for a rectangle?
Its diagonals are perpendicular.
Fill in the blank in the following, so as to make the statement true:
A rectangle is a parallelogram in which .....
The sides of a rectangle are in the ratio 4 : 5. Find its sides if the perimeter is 90 cm.
Draw a rectangle whose one side measures 8 cm and the length of each of whose diagonals is 10 cm.
Draw a square whose each side measures 4.8 cm.
Prove that quadrilateral formed by the intersection of angle bisectors of all angles of a parallelogram is a rectangle.
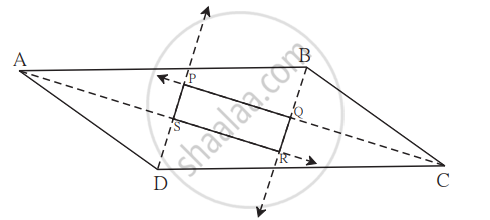
Show that the bisectors of angles of a parallelogram form a rectangle
If diagonals of a quadrilateral are equal, it must be a rectangle.
Every trapezium is a rectangle.
