Advertisements
Advertisements
प्रश्न
Diagonals of a parallelogram ABCD intersect at O. AL and CM are drawn perpendiculars to BD such that L and M lie on BD. Is AL = CM? Why or why not?
उत्तर

\[\text{ In } \Delta AOL \text{ and } \Delta CMO: \]
\[\angle AOL = \angle COM( \text{ vertically opposite angle }) . . . . (i)\]
\[\angle ALO = \angle CMO = 90° (\text{ each right angle }) . . . . . (ii)\]
\[\text{ Using angle sum property }: \]
\[\angle AOL + \angle ALO + \angle LAO = 180° . . . . . . . . . . (iii)\]
\[\angle COM + \angle CMO + \angle OCM = 180°. . . . . . (iv)\]
\[\text{ From equations } (iii) \text{ and } (iv): \]
\[\angle AOL + \angle ALO + \angle LAO = \angle COM + \angle CMO + \angle OCM\]
\[\angle LAO = \angle OCM (\text{ from equations (i) and } (ii) )\]
\[In \Delta AOL \text{ and }\Delta CMO: \]
\[\angle ALO = \angle CMO (\text{ each right angle })\]
\[AO = OC (\text{ diagonals of a parallelogram bisect each other })\]
\[\angle LAO = \angle OCM (\text{ proved above })\]
\[\text{ So }, \Delta AOL \text{ is congruent to } \Delta CMO (SAS) . \]
\[ \Rightarrow AL = CM [cpct]\]
APPEARS IN
संबंधित प्रश्न
Explain why a rectangle is a convex quadrilateral.
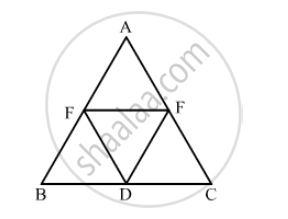
In the following figure, BDEF and DCEF are each a parallelogram. Is it true that BD = DC? Why or why not?
Which of the following statement is true for a rectangle?
Its diagonals bisect each other.
State with Reason Whether the Following Statement is ‘True’ Or ‘False’.
Every rectangle is a parallelogram.
If diagonal of a rectangle is 26 cm and one side is 24 cm, find the other side.
The following figure is a rectangle in which x: y = 3: 7; find the values of x and y.
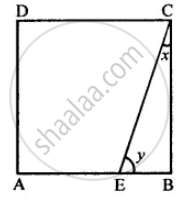
The interior angle made by the side in a parallelogram is 90° then the parallelogram is a
If the diagonals of a quadrilateral are equal and bisect each other, then the quadrilateral is a ______.
In rectangle READ, find ∠EAR, ∠RAD and ∠ROD
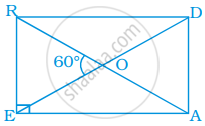
Construct a rectangle whose one side is 3 cm and a diagonal equal to 5 cm.
