Advertisements
Advertisements
Question
The sides of a rectangle are in the ratio 2 : 3, and its perimeter is 20 cm. Draw the rectangle.
Advertisements
Solution
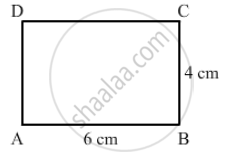
\[\text{ Let the side be x cm and y cm } . \]
\[\text{ So, we have }: \]
\[ 2\left( x + y \right) = 20 \]
\[\text{ Sides are in the ratio 2: 3 } . \]
\[ \therefore y = \frac{3x}{2}\]
\[\text{ Putting the value of y }: \]
\[2\left( x + \frac{3x}{2} \right) = 20 \]
\[\frac{2x + 3x}{2} = 10\]
\[5x = 20\]
\[x = 4\]
\[ \therefore y = \frac{3 \times 4}{2} = 6\]
\[\text{ Thus, sides of the rectangle will be 4 cm and 6 cm } . \]
\[\text{ ABCD is the rectangle having sides 4 cm and 6 cm } .\]
RELATED QUESTIONS
Name the quadrilaterals whose diagonals are equal
Which of the following statement is true for a rectangle?
It has two pairs of equal sides.
Which of the following statement is true for a rectangle?
Its diagonals are equal and perpendicular, and bisect each other.
Which of the following statement true for a square?
Its diagonals bisect each other at right angle.
Which of the following statement true for a square?
Its diagonals are equal to its sides.
Using opposite angles test for parallelogram, prove that every rectangle is a parallelogram.
A parallelogram PQRS is constructed with sides QR = 6 cm, PQ = 4 cm and ∠PQR = 90°. Then PQRS is a ______.
If diagonals of a quadrilateral are equal, it must be a rectangle.
Every parallelogram is a rectangle.
A line l is parallel to line m and a transversal p intersects them at X, Y respectively. Bisectors of interior angles at X and Y interesct at P and Q. Is PXQY a rectangle? Given reason.
