Advertisements
Advertisements
Question
If diagonal of a rectangle is 26 cm and one side is 24 cm, find the other side.
Solution
Suppose ABCD is a rectangle.
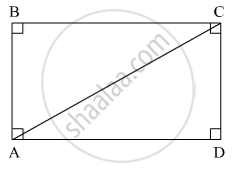
Here, segment AC is a diagonal and segment AD is one side of the rectangle ABCD.
l(AC) = 26 cm and l(AD) = 24 cm.
In right ∆ACD,
l(AC)2 = l(AD)2 + l(CD)2 ...(Pythagoras theorem)
⇒ l(CD)2 = l(AC)2 − l(AD)2
⇒ l(CD)2 = (26)2 − (24)2
⇒ l(CD)2 = 676 − 576 = 100
⇒ l(CD) = `sqrt(100)` = 10 cm
Thus, the other side of the rectangle is 10 cm.
RELATED QUESTIONS
Name the quadrilaterals whose diagonals are equal
Diagonals of a parallelogram ABCD intersect at O. AL and CM are drawn perpendiculars to BD such that L and M lie on BD. Is AL = CM? Why or why not?
Which of the following statement is true for a rectangle?
It has all its sides of equal length.
Which of the following statement is true for a rectangle?
Its diagonals are equal.
Which of the following statement is true for a rectangle?
Its diagonals are perpendicular and bisect each other.
Fill in the blank of the following, so as to make the statement true:
A square is a rectangle in which .....
A window frame has one diagonal longer than the other. Is the window frame a rectangle? Why or why not?
Draw a square whose each side measures 4.8 cm.
Using opposite angles test for parallelogram, prove that every rectangle is a parallelogram.
A rectangular MORE is shown below:
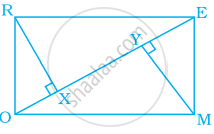
Answer the following questions by giving appropriate reason.
- Is RE = OM?
- Is ∠MYO = ∠RXE?
- Is ∠MOY = ∠REX?
- Is ΔMYO ≅ ΔRXE?
- Is MY = RX?
