Advertisements
Advertisements
Question
Lengths of diagonals of a rhombus ABCD are 16 cm and 12 cm. Find the side and perimeter of the rhombus.
Solution
ABCD is a rhombus.
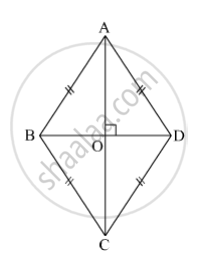
Here, segment AC and segment BD are the diagonals of the rhombus ABCD.
l(AC) = 16 cm and l(BD) = 12 cm.
Diagonals of a rhombus are perpendicular bisectors of each other.
∴ m∠AOD = 90°
Also, l(OA) = `1/2l(AC) = 1/2 xx 16 = 8 cm`
l(OD) = `1/2l(BD) = 1/2`× 12 = 6 cm
In right ∆AOD,
l(AD)2 = l(OA)2 + l(OD)2 ...[Pythagoras theorem]
⇒ l(AD)2 = (8)2 + (6)2
⇒ l(AD)2 = 64 + 36 = 100
⇒ l(AD) = \[\sqrt{100}\] = 10 cm
All sides of a rhombus are equal.
∴ Perimeter of the rhombus ABCD = 4 × Side of a rhombus = 4 × 10 = 40 cm
Thus, the side and perimeter of the rhombus are 10 cm and 40 cm, respectively.
RELATED QUESTIONS
All rhombuses are parallelograms.
Which of the following statement is true for a rhombus?
It has all its sides of equal lengths.
Which of the following statement is true for a rhombus?
It is a parallelogram.
Fill in the blank, in the following, so as to make the statement true:
The diagonals of a rhombus ...... each other at ...... angles.
ABCD is a rhombus. If ∠ACB = 40°, find ∠ADB.
Draw a rhombus, having each side of length 3.5 cm and one of the angles as 40°.
Draw a rhombus ABCD, if AB = 6 cm and AC = 5 cm.
In rhombus ABCD;
(i) if ∠A = 74° ; find ∠B and ∠C.
(ii) if AD = 7.5 cm ; find BC and CD.
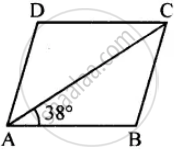
ABCD is a rhombus. If ∠BAC = 38°, find :
(i) ∠ACB
(ii) ∠DAC
(iii) ∠ADC.
If the adjacent sides of a parallelogram are equal then parallelogram is a ______.
