Advertisements
Advertisements
Question
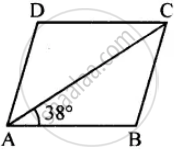
ABCD is a rhombus. If ∠BAC = 38°, find :
(i) ∠ACB
(ii) ∠DAC
(iii) ∠ADC.
Solution
ABCD is Rhombus (Given)
AB = BC
∠BAC = ∠ACB (∠s opp. to equal sides)
But ∠BAC = 38° (Given)
∠ACB = 38°
In ∆ABC,
∠ABC + ∠BAC + ∠ACB = 180°
∠ABC + 38°+ 38° = 180°
∠ABC = 180° – 76° = 104°
But ∠ABC = ∠ADC (opp. ∠s of rhombus)
∠ADC = 104°
∠DAC = ∠DCA ( AD = CD)
∠DAC = `1/2` [180° - 104°]
∠DAC = `1/2 xx` 76° = 38°
Hence (i) ∠ACB = 38° (ii) ∠DAC = 38° (iii) ∠ADC = 104° Ans.
APPEARS IN
RELATED QUESTIONS
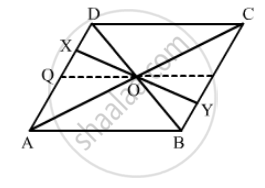
Diagonals of parallelogram ABCD intersect at O as shown in the following fegure. XY contains O, and X, Y are points on opposite sides of the parallelogram. Give reasons for each of the following:
(i) OB = OD
(ii) ∠OBY = ∠ODX
(iii) ∠BOY = ∠DOX
(iv) ∆BOY ≅ ∆DOX
Now, state if XY is bisected at O.
Which of the following statement is true for a rhombus?
It has two pairs of parallel sides.
Fill in the blank, in the following, so as to make the statement true:
A rhombus is a parallelogram in which ......
In a rhombus PQRS if PQ = 7.5 cm then find QR. If ∠QPS = 75° then find the measure of ∠PQR and ∠SRQ.
A quadrilateral whose all sides are equal, opposite angles are equal and the diagonals bisect each other at right angles is a ______.
In the figure, BEST is a rhombus, Then the value of y – x is ______.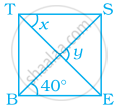
A rhombus is a parallelogram in which ______ sides are equal.
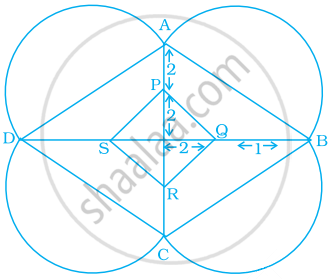
A Rangoli has been drawn on a flor of a house. ABCD and PQRS both are in the shape of a rhombus. Find the radius of semicircle drawn on each side of rhombus ABCD.
Construct a rhombus whose side is 5 cm and one angle is of 60°.
Construct a rhombus CLUE in which CL = 7.5 cm and LE = 6 cm.
