Advertisements
Advertisements
Question
ABCD is a rhombus. If ∠ACB = 40°, find ∠ADB.
Advertisements
Solution
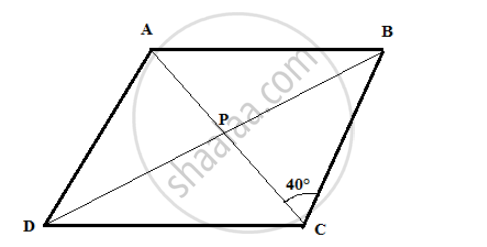
\[\text{ In a rhombus, the diagonals are perpendicular } . \]
\[ \therefore \angle BPC = 90°\]
\[\text{ From ∆ BPC, the sum of angles is }180° . \]
\[ \therefore \angle CBP + \angle BPC + \angle PBC = 180°\]
\[\angle CBP = 180° - \angle BPC - \angle PBC\]
\[\angle CBP = 180° - 40° - 90° = 50° \]
\[\angle ADB = \angle CBP = 50°(\text{ alternate angle })\]
RELATED QUESTIONS
Name the quadrilaterals whose diagonals are perpendicular bisectors of each other
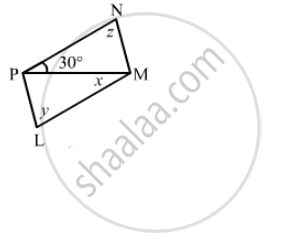
The following figure is parallelogram. Find the degree value of the unknown x, y, z.
Can the following figure be parallelogram. Justify your answer.
In the following figure GUNS and RUNS are parallelogram. Find x and y.
Which of the following statement is true for a rhombus?
Its diagonals bisect each other at right angles.
Which of the following statement is true for a rhombus?
It is a square.
The diagonals of a quadrilateral are perpendicular to each other. Is such a quadrilateral always a rhombus? If your answer is 'No', draw a figure to justify your answer.
A quadrilateral whose all sides are equal, opposite angles are equal and the diagonals bisect each other at right angles is a ______.
ABCD is a rhombus such that the perpendicular bisector of AB passes through D. Find the angles of the rhombus.
Hint: Join BD. Then ∆ABD is equilateral.
Construct a rhombus whose side is 5 cm and one angle is of 60°.
