Advertisements
Advertisements
Question
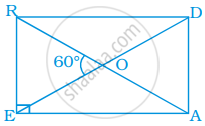
In rectangle READ, find ∠EAR, ∠RAD and ∠ROD
Advertisements
Solution
Given, READ is a rectangle in which the diagonals ED and AR intersect at O.
We have, ROA is a straight line
So, ∠ROE + ∠EOA = 180°
`\implies` ∠EOA = 180° – 60°
`\implies` ∠EOA = 120° ...(i)
In ΔOEA, OE = OA ...[∵ Diagonals of a rectangle are equal and they bisect each other]
`\implies` ∠OEA = ∠OAE = x ...(Say)
And ∠OEA + ∠EAO + ∠AOE = 180° ...[Angle sum property]
`\implies` x + x + 120° = 180° ...[From (i)]
`\implies` 2x = 60°
`\implies` x = 30°
∴ ∠EAO = 30°
`\implies` ∠EAR = 30°
Now, ∠RAD = ∠EAD – ∠EAR
= 90° – 30° ...[∵ ∠A = 90°]
= 60°
As ∠EOA = ∠DOR = 120° ...[∵ Vertically opposite angles]
Hence, ∠EAR = 30°, ∠RAD = 60° and ∠ROD = 120°
APPEARS IN
RELATED QUESTIONS
In a parallelogram ABCD, the diagonals bisect each other at O. If ∠ABC = 30°, ∠BDC = 10° and ∠CAB = 70°. Find:
∠DAB, ∠ADC, ∠BCD, ∠AOD, ∠DOC, ∠BOC, ∠AOB, ∠ACD, ∠CAB, ∠ADB, ∠ACB, ∠DBC and ∠DBA.
In Fig. 17.29, suppose it is known that DE = DF. Then, is ΔABC isosceles? Why or why not?
Which of the following statement are true for a square?
It has all its sides of equal length.
Fill in the blank of the following, so as to make the statement true:
A square is a rectangle in which .....
The sides of a rectangle are in the ratio 4 : 5. Find its sides if the perimeter is 90 cm.
If the adjacent angles of a parallelogram are equal, then the parallelogram is a ______.
Rectangle is a regular quadrilateral.
If diagonals of a quadrilateral are equal, it must be a rectangle.
Every rectangle is a trapezium.
A playground is in the form of a rectangle ATEF. Two players are standing at the points F and B where EF = EB. Find the values of x and y.
