Advertisements
Advertisements
प्रश्न
A magnetic field B is confined to a region r ≤ a and points out of the paper (the z-axis), r = 0 being the centre of the circular region. A charged ring (charge = Q) of radius b, b > a and mass m lies in the x-y plane with its centre at the origin. The ring is free to rotate and is at rest. The magnetic field is brought to zero in time ∆t. Find the angular velocity ω of the ring after the field vanishes.
उत्तर
According to the law of EMI, when magnetic field changes in the circuit, then magnetic flux linked with the circuit also changes and this changing magnetic flux leads to an induced emf in the circuit.
Here, magnetic field decreases which causes induced emf and hence, electric field around the ring. The torque experienced by the ring produces change in angular momentum.
As the magnetic field is brought to zero in time At, the magnetic flux linked with the ring also reduces from maximum to zero. This, in turn, induces an emf in ring as discussed above. The induces emf causes the induced electric field E around the ring.
By the relation between electric field and potential we get,
The induced emf = Electric field E × (2πb) (Because V = E × d) ......(i)
By Faraday's law of EMI
`|ε| = (dphi)/(dt) = A (dB)/(dt)`
`|ε| = (Bpia^2)/(Δt) S` ......(ii)
From equation (i) and (ii), we have
`2pibE = ε = (Bpia^2)/(Δt)`
As we know the electric force experienced by the charged ring, `F_e = QE`. This force try to rotate the coil, and the torque is given by
Torque = b × Force
τ = `QEb = Q[(Bpia^2)/(2piΔt)]b`
⇒ τ = `Q (Ba^2)/(2Δt)`
If ΔL is the change in angular momentum,
ΔL = Torque × Δt = `Q (Ba^2)/2`
Since, initial angular momentum = 0
And Torque × Δt = Change in angular momentum
Final angular momentum = `mb^2ω = (QBa^2)/2`
Where, mb2 = I (moment of inertia of ring)
`ω = (QBa^2)/(2mb^2)`
On rearranging the terms, we have the required expression of angular speed.
APPEARS IN
संबंधित प्रश्न
A circular coil of radius 8.0 cm and 20 turns is rotated about its vertical diameter with an angular speed of 50 rad s−1 in a uniform horizontal magnetic field of magnitude 3.0 × 10−2 T. Obtain the maximum and average emf induced in the coil. If the coil forms a closed loop of resistance 10 Ω, calculate the maximum value of current in the coil. Calculate the average power loss due to Joule heating. Where does this power come from?
A jet plane is travelling towards west at a speed of 1800 km/h. What is the voltage difference developed between the ends of the wing having a span of 25 m, if the Earth’s magnetic field at the location has a magnitude of 5 × 10−4 T and the dip angle is 30°.
State Faraday's first law of electrolysis.
A .0.5m long solenoid of 10 turns/cm has area of cross-section 1cm2 . Calculate the voltage induced across its ends if the current in the solenoid is changed from 1A to 2A in 0.1s.
The two rails of a railway track, insulated from each other and the ground, are connected to millivoltmeter. What is the reading of the millivoltmeter when a train passes at a speed of 180 km/hr along the track, given that the vertical component of earth’s magnetic field is 0.2 × 10–4 wb/m2 and rails are separated by 1 metre ______.
According to Faraday's law of electromagnetic induction, ______
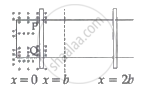
The arm PQ of a rectangular conductor is moving from x = 0 to x = 2b outwards and then inwards from x = 2b to x = 0 as shown in the figure. A uniform magnetic field perpendicular to the plane is acting from x = 0 to x = b. Identify the graph showing the variation of different quantities with distance.
Two coils C1 and C2 are placed close to each other. The magnetic flux Φ2 linked with coil C2 varies with the current I1 flowing in coil C1 as shown in the figure. Find

- The mutual inductance of the arrangement, and
- The rate of change of current `((dI_1)/(dt))` will induce an emf of 100V in coil C2.
How much charge in terms of Faraday is required for the reduction of 1 mol of Cu2+ to Cu?
