Advertisements
Advertisements
प्रश्न
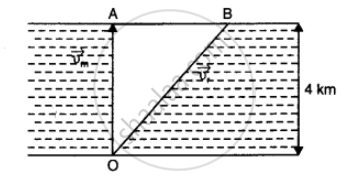
A man can swim with a speed of 4.0 km/h in still water. How long does he take to cross a river 1.0 km wide if the river flows steadily at 3.0 km/h and he makes his strokes normal to the river current? How far down the river does he go when he reaches the other bank?
उत्तर १
Hera, `vecV_m = 4 km h^(-1), vecv_r = 3 km h^(-1)`
OA = 1 km
Let t = time taken by man to reach the other bank
then `t = (OA)/v_m = 1/4 = 0.25 h`
Distance `AB = v_t xx t =3 xx 0.25 = 0.75 km.`
उत्तर २
Speed of the man, vm = 4 km/h
Width of the river = 1 km
Time taken to cross the river = `"Width of river"/"Speed of man"`
`=1/4h = 1/4 xx 60 = 15 min`
Speed of the river, vr = 3 km/h
Distance covered with flow of the river = vr × t
`= 3 xx 1/4 = 3/4` km
`=3/4 xx 1000 = 750 m`
APPEARS IN
संबंधित प्रश्न
Show that for a projectile the angle between the velocity and the x-axis as a function of time is given by
`theta(t) =tan^(-1) ((v_(0y) - "gt")/v_(o x))`
A ball rolls of the top of the stairway with a horizontal velocity u ms−1. If the steps are h m high and b m wide, the ball will hit the edge of the nth step, if:
A car starts from rest and accelerates at 5 m/s2. At t = 4 s, a ball is dropped out of a window by a person sitting in the car. What is the velocity and acceleration of the ball at t = 6 s? (Take g = 10 m/s2)
A particle moving in a circle of radius R with a uniform speed takes a time T to complete one revolution.
If this particle were projected with the same speed at an angle ‘θ’ to the horizontal, the maximum height attained by it equals 4R. The angle of projection, θ, is then given by ______.
A fighter plane is flying horizontally at an altitude of 1.5 km with speed 720 km/h. At what angle of sight (w.r.t. horizontal) when the target is seen, should the pilot drop the bomb in order to attack the target?
A particle is projected in air at an angle β to a surface which itself is inclined at an angle α to the horizontal (Figure).
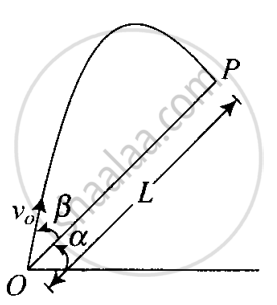
- Find an expression of range on the plane surface (distance on the plane from the point of projection at which particle will hit the surface).
- Time of flight.
- β at which range will be maximum.
If T be the total time of flight of a current of water and H be the maximum height attained by it from the point of projection, then HIT will be : (u = projection velocity, e = projection angle)
If a baseball player can throw a ball at maximum distance = d over a ground, the maximum vertical height to which he can throw it, will be (Ball has same initial speed in each case):
A circular disc of radius r = 5m is rotating in horizontal plane about y-axis. Y-axis is vertical axis passing through the centre of disc and x-z is the horizontal plane at ground. The height of disc above ground is h = 5 m. Small particles are ejecting from disc in horizontal direction with speed 12 m/s from the circumference of disc then the distance of these particles from origin when they hits the x-z plane is:
The trajectories of the two projectiles are shown in the figure. Let T1 and T2 be the time periods and u1 and u2 be their speeds of projection. Then ______.