Advertisements
Advertisements
प्रश्न
A metallic sheet is of the rectangular shape with dimensions 48cm x 36cm. From each one of its corners, a square of 8cm is cutoff. An open box is made of the remaining sheet. Find the volume of the box.
उत्तर
Given that
Dimensions of metallic sheet:
Length (l) = 48cm
Breadth (b) = 36cm
Side (S) of each square = 8cm.
Now,
Area of metallic sheet
= l x b
= 48 x 36
= 1728cm2
Area of 1 square
= S x S
= 8 x 8
= 64cm2
∴ Area of 4 square
= 64 x 4
= 256cm2
Thus, remaining area in the sheet after reducing the area of 4 squares:
Remaining area
= 1728 - 256
= 1472cm2 ................................(1)
Since 8cm square is cut off from all sides, we get the dimensions of open box as:
Length (l) = 48 - 16 = 32cm
Breadth (b) = 36 - 16 = 20cm
Area of the box = L.S.A of the box + area of base of the box
1472 = {2 x h x (l + b)} + (l x b) ...[From (1)]
1472 = {2 x h x (32 + 20)} + (32 x 20)
1472 = {2h x 52} + 640
1472 = 104h + 640
104h = 1472 - 640
h = `(832)/(104)`
i.e., height(h) = 8cm
Thus,
volume of the box
= l x b x h
= 32 x 20 x 8
= 5120cm3.
APPEARS IN
संबंधित प्रश्न
A plastic box 1.5 m long, 1.25 m wide and 65 cm deep, is to be made. It is to be open at the top. Ignoring the thickness of the plastic sheet, determine:
(i) The area of the sheet required for making the box.
(ii) The cost of sheet for it, if a sheet measuring 1 m2 costs Rs 20.
The dimensions of a rectangular box are in the ratio of 2 : 3 : 4 and the difference between the cost ofcovering it with sheet of paper at the rates of Rs. 8 and Rs. 9.50 per m2 is Rs.1248. Find the dimensions of the box.
Ravish wanted to make a temporary shelter for his car by making a box-like structure with tarpaulin that covers all the four sides and the top of the car ( with the front face as a flap which can be rolled up). Assuming that the stitching margins are very small, and therefore negligible, how much tarpaulin would be required to make the shelter of height 2.5 m with
base dimensions 4 m × 3m?
Find the height of a cuboid of volume 100 cm3, whose length and breadth are 5 cm and 4 cm respectively.
A cuboidal block of solid iron has dimensions 50 cm, 45 cm and 34 cm. How many cuboids of size 5 cm by 3 cm by 2 cm can be obtained from this block? Assume cutting causes no wastage.
If V is the volume of a cuboid of dimensions x, y, z and A is its surface area, then `A/V`
The internal length, breadth, and height of a closed box are 1 m, 80 cm, and 25 cm. respectively. If its sides are made of 2.5 cm thick wood; find :
(i) the capacity of the box
(ii) the volume of wood used to make the box.
The floor of a rectangular hall has a perimeter 250 m. If the cost of painting the four walls at the rate of ₹ 10 per m2 is ₹ 15,000, find the height of the hall.
The length, breadth, and height of a rectangular solid are in the ratio 6 : 4 :3. If the total surface area is 1728 cm2. Find its dimensions.
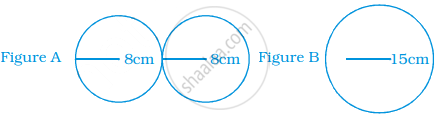
Below are the drawings of cross sections of two different pipes used to fill swimming pools. Figure A is a combination of 2 pipes each having a radius of 8 cm. Figure B is a pipe having a radius of 15 cm. If the force of the flow of water coming out of the pipes is the same in both the cases, which will fill the swimming pool faster?