Advertisements
Advertisements
प्रश्न
A model of a ship is made to a scale of 1:500. Find: The volume of the model when the volume of the ship is 1km3
उत्तर
Scale = 1:500
Volume of the ship = 1km3
`"volume(model)"/"volume(ship)"` = Scale
`"volume(model)"/(1 xx (1000)^3) = (1)/(500)^3`
Volume(model) = `(1000000000)/(125000000)`
Volume(model) = 8m3.
APPEARS IN
संबंधित प्रश्न
D is a point on the side BC of ∆ABC such that ∠ADC = ∠BAC. Prove that` \frac{"CA"}{"CD"}=\frac{"CB"}{"CA"} or "CA"^2 = "CB" × "CD".`
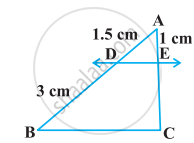
See the given figure. DE || BC. Find EC.
E and F are the points in sides DC and AB respectively of parallelogram ABCD. If diagonal AC
and segment EF intersect at G; prove that:
Prove that the area of the triangle BCE described on one side BC of a square ABCD as base is one half of the area of similar triangle ACF described on the diagonal AC as base.
In the adjoining figure. BC is parallel to DE, area of ΔABC = 25 sq cm, area of trapezium BCED = 24 sq cm, DE = 14 cm. Calculate the length of BC.
ΔABC has been reduced by a scale factor 0.6 to ΔA'B'C'/ Calculate: Length of AB, if A'B' = 5.4cm
The scale of a map is 1 : 50000. The area of a city is 40 sq km which is to be represented on the map. Find: The area of land represented on the map.
D is the mid point of side BC and AE ⊥ BC. If BC = a, AC = b, AB = c, ED = x, AD = p and AE = h, prove that c2 = `"p"^2 - "a"x + "a"^2/4`
Areas of two similar triangles are 225 cm2 and 81 cm2. If side of smaller triangle is 12 cm, find corresponding side of major triangle.
In a square of side 10 cm, its diagonal = ______.
