Advertisements
Advertisements
प्रश्न
A point object is placed on the principal axis of a convex spherical surface of radius of curvature R, which separates the two media of refractive indices n1 and n2 (n2 > n1). Draw the ray diagram and deduce the relation between the object distance (u), image distance (v) and the radius of curvature (R) for refraction to take place at the convex spherical surface from rarer to denser medium.
उत्तर
A refracting surface which forms a part of a sphere of transparent refracting material is called a spherical refracting surface.
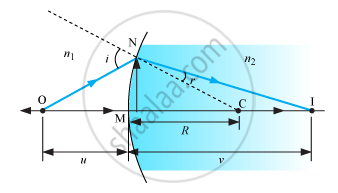
The above figure shows the geometry of formation of image I of an object O and the principal axis of a spherical surface with centre of curvature Cand radius of curvature R.
Assumptions:
(i) The aperture of the surface is small compared to other distance involved.
(ii) NM will be taken to be nearly equal to the length of the perpendicular from the point N on the principal axis.
`tan ∠NOM = (MN)/(OM)`
`tan ∠NCM =(MN)/(MC) `
`tan ∠NIM = (MN)/(MI)`
For ΔNOC, i is the exterior angle.
∴ i = ∠NOM + ∠NCM
`i = (MN)/(OM)+(MN)/(MC) ........... (1)`
Similarly, r = ∠NCM − ∠NIM
`i.e.,r = (MN)/(MC) - (MN)/(MI) ......(2) `
By Snell’s law,
n1sini = n2sinr
For small angles,
n1i= n2 r
Substituting the values of i and r from equations (i) and (ii), we obtain
`n_1( (MN)/(OM)+(MN)/(MC))= n_2((MN)/(MC) - (MN)/(MI)) `
or,`n_1/(OM) +n_2/(MI)= (n_2 - n_1)/(MC) ........... (3)`
Applying new Cartesian sign conventions,
OM = − u, MI = + v, MC = + R
Substituting these in equation (3), we obtain
`n_2/v-n_1/u = (n_2-n_1)/R ...... (4)`
Above equation is the required equation.
APPEARS IN
संबंधित प्रश्न
Find the electric field intensity due to a uniformly charged spherical shell at a point (i) outside the shell. Plot the graph of electric field with distance from the centre of the shell.
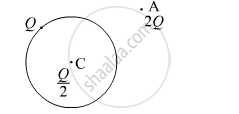
A thin metallic spherical shell of radius R carries a charge Q on its surface. A point charge`Q/2` is placed at its centre C and an other charge +2Q is placed outside the shell at a distance x from the centre as shown in the figure. Find (i) the force on the charge at the centre of shell and at the point A, (ii) the electric flux through the shell.
Find the ratio of the potential differences that must be applied across the parallel and series combination of two capacitors C1 and C2 with their capacitances in the ratio 1 : 2 so that the energy stored in the two cases becomes the same.
An infinitely large thin plane sheet has a uniform surface charge density +σ. Obtain the expression for the amount of work done in bringing a point charge q from infinity to a point, distant r, in front of the charged plane sheet.
Using Gauss’ law deduce the expression for the electric field due to a uniformly charged spherical conducting shell of radius R at a point
(i) outside and (ii) inside the shell.
Plot a graph showing variation of electric field as a function of r > R and r < R.
(r being the distance from the centre of the shell)
A charge Q is uniformly distributed on a spherical shell. What is the field at the centre of the shell? If a point charge is brought close to the shell, will the field at the centre change? Does your answer depend on whether the shell is conducting or non-conducting?
A spherical shell made of plastic, contains a charge Q distributed uniformly over its surface. What is the electric field inside the shell? If the shell is hammered to deshape it, without altering the charge, will the field inside be changed? What happens if the shell is made of a metal?
A positive point charge Q is brought near an isolated metal cube.
A large non-conducting sheet M is given a uniform charge density. Two uncharged small metal rods A and B are placed near the sheet as shown in the following figure.
(a) M attracts A.
(b) M attracts B.
(c) A attracts B.
(d) B attracts A.

Find the flux of the electric field through a spherical surface of radius R due to a charge of 10−7 C at the centre and another equal charge at a point 2R away from the centre in the following figure.

