Advertisements
Advertisements
प्रश्न
Using Gauss’ law deduce the expression for the electric field due to a uniformly charged spherical conducting shell of radius R at a point
(i) outside and (ii) inside the shell.
Plot a graph showing variation of electric field as a function of r > R and r < R.
(r being the distance from the centre of the shell)
उत्तर
Electric Field Due To A Uniformly Charged Thin Spherical Shell:
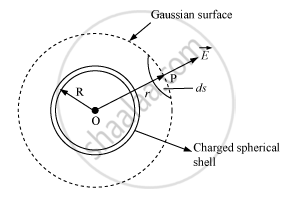
(i) When point P lies outside the spherical shell:
Suppose that we have to calculate electric field at the point P at a distance r (r > R) from its centre. Draw the Gaussian surface through point P so as to enclose the charged spherical shell. The Gaussian surface is a spherical shell of radius r and centre O.
Let `vecE`be the electric field at point P. Then, the electric flux through area element vecdsis given by,
`dphi = vecE.vecds`
Since `vecds` s also along normal to the surface,
dΦ = E ds
∴ Total electric flux through the Gaussian surface is given by,
`phi = oint_s Eds = Eoint_s ds`
Now,
`oint ds = 4pir^2`
`therefore phi= E xx 4pir^2 ..... (1)`
Since the charge enclosed by the Gaussian surface is q, according to Gauss theorem,
`phi = q/epsi_0 ......(2)`
From equations (i) and (ii), we obtain
`E xx 4pir^2q/epsi_o`
`E = 1/(4piepsi_0).q/r^2` (for r>R)
(ii) When point P lies inside the spherical shell:
In such a case, the Gaussian surface encloses no charge.
According to Gauss law,
E × 4πr2 = 0
i.e., = E = 0 (r < R)
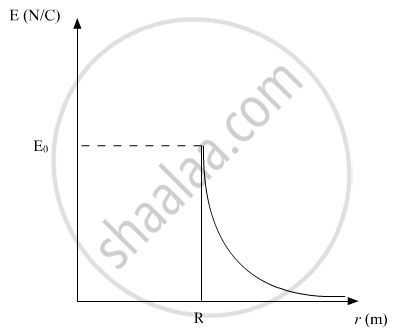
Graph showing the variation of electric field as a function of r:
APPEARS IN
संबंधित प्रश्न
Find the electric field intensity due to a uniformly charged spherical shell at a point (i) outside the shell. Plot the graph of electric field with distance from the centre of the shell.
Find the electric field intensity due to a uniformly charged spherical shell at a point (ii) inside the shell. Plot the graph of electric field with distance from the centre of the shell.
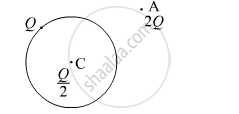
A thin metallic spherical shell of radius R carries a charge Q on its surface. A point charge`Q/2` is placed at its centre C and an other charge +2Q is placed outside the shell at a distance x from the centre as shown in the figure. Find (i) the force on the charge at the centre of shell and at the point A, (ii) the electric flux through the shell.
Two large, thin metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and of magnitude 17.0 × 10−22 C/m2. What is E:
- in the outer region of the first plate,
- in the outer region of the second plate, and
- between the plates?
How is the field directed if (i) the sheet is positively charged, (ii) negatively charged?
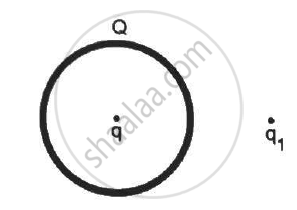
A thin, metallic spherical shell contains a charge Q on it. A point charge q is placed at the centre of the shell and another charge q1 is placed outside it as shown in the following figure . All the three charges are positive. The force on the charge at the centre is ____________.
A circular wire-loop of radius a carries a total charge Q distributed uniformly over its length. A small length dL of the wire is cut off. Find the electric field at the centre due to the remaining wire.
“A uniformly charged conducting spherical shell for the points outside the shell behaves as if the entire charge of the shell is concentrated at its centre”. Show this with the help of a proper diagram and verify this statement.
