Advertisements
Advertisements
प्रश्न
“A uniformly charged conducting spherical shell for the points outside the shell behaves as if the entire charge of the shell is concentrated at its centre”. Show this with the help of a proper diagram and verify this statement.
उत्तर
A shell of radius R is carrying uniformly distributed charge of charge density σ.
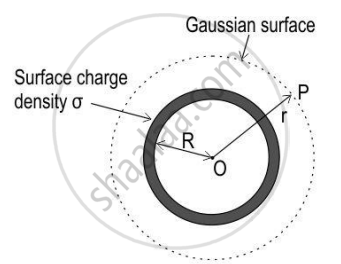
Electric field E at point P at a distance r from the centre of a uniformly charged spherical shell:
Consider a Gaussian surface to be a sphere of radius r >R and with centre O, passing through P.
Using the principles of spherical symmetry, E and area vector ΔS at every point are parallel, flux through each of the area element.
ΔΦ = E. ΔS
Summing over all ΔS,
Φ = E × 4 π R2
The charge enclosed is σ × 4 π R2
By Gauss’ law,
`"E" xx 4 pi "r"^2 = "q"/ε_o = (σ "X" 4pi "R"^2)/ε_o`
`"E" = (σ "R"^2)/(ε_o "r"^2) = "q"/(4 pi ε_o "r"^2)`
This formula is exactly similar to the field produced by a point charge q placed at the centre O, that is, as per Coulomb’s law.
APPEARS IN
संबंधित प्रश्न
Two large, thin metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and of magnitude 17.0 × 10−22 C/m2. What is E:
- in the outer region of the first plate,
- in the outer region of the second plate, and
- between the plates?
An infinitely large thin plane sheet has a uniform surface charge density +σ. Obtain the expression for the amount of work done in bringing a point charge q from infinity to a point, distant r, in front of the charged plane sheet.
Using Gauss's law in electrostatics, deduce an expression for electric field intensity due to a uniformly charged infinite plane sheet. If another identical sheet is placed parallel to it, show that there is no electric field in the region between the two sheets ?
A point object is placed on the principal axis of a convex spherical surface of radius of curvature R, which separates the two media of refractive indices n1 and n2 (n2 > n1). Draw the ray diagram and deduce the relation between the object distance (u), image distance (v) and the radius of curvature (R) for refraction to take place at the convex spherical surface from rarer to denser medium.
A small conducting sphere of radius 'r' carrying a charge +q is surrounded by a large concentric conducting shell of radius Ron which a charge +Q is placed. Using Gauss's law, derive the expressions for the electric field at a point 'x'
(i) between the sphere and the shell (r < x < R),
(ii) outside the spherical shell.
Using Gauss’s law, prove that the electric field at a point due to a uniformly charged infinite plane sheet is independent of the distance from it.
A charge Q is uniformly distributed on a spherical shell. What is the field at the centre of the shell? If a point charge is brought close to the shell, will the field at the centre change? Does your answer depend on whether the shell is conducting or non-conducting?
A large non-conducting sheet M is given a uniform charge density. Two uncharged small metal rods A and B are placed near the sheet as shown in the following figure.
(a) M attracts A.
(b) M attracts B.
(c) A attracts B.
(d) B attracts A.

Find the flux of the electric field through a spherical surface of radius R due to a charge of 10−7 C at the centre and another equal charge at a point 2R away from the centre in the following figure.

A circular wire-loop of radius a carries a total charge Q distributed uniformly over its length. A small length dL of the wire is cut off. Find the electric field at the centre due to the remaining wire.
