Advertisements
Advertisements
प्रश्न
A rectangular sheet of paper is rolled in two different ways to form two different cylinders. Find the volume of cylinders in each case if the sheet measures 44 cm × 33 cm.
उत्तर
 |
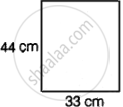 |
| (i) | (ii) |
In 1st case,
If sheet rolled along 44 cm
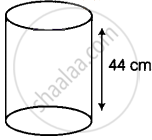
⇒ 2πr = 44 cm
∴ `r = 44/(2pi)`
= `44/2 xx 7/22`
= 7 cm
In 2nd case,
If sheet rolled along 33 cm
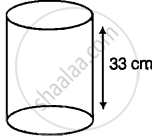
⇒ 2πr = 33
∴ `r = 33/(2pi)`
= `(33 xx 7)/(2 xx 22)`
= `(3 xx 7)/(2 xx 2)`
= `21/4` cm
APPEARS IN
संबंधित प्रश्न
A soft drink is available in two packs − (i) a tin can with a rectangular base of length 5 cm and width 4 cm, having a height of 15 cm and (ii) a plastic cylinder with circular base of diameter 7 cm and height 10 cm. Which container has greater capacity and by how much?
`["Assume "pi=22/7]`
A vessel is a hollow cylinder fitted with a hemispherical bottom of the same base. The depth of the cylindrical part is `4 2/3` m and the diameter of hemisphere is 3.5 m. Calculate the capacity and the internal surface area of the vessel.
The height and the radius of the base of a cylinder are in the ratio 3 : 1. If it volume is 1029 π cm3; find it total surface area.
A cylinder of circumference 8 cm and length 21 cm rolls without sliding for `4 1/2` seconds at the rate of 9 complete rounds per second. Find the area covered by the cylinder in `4 1/2` seconds.
A closed cylindrical tank, made of thin ironsheet, has diameter = 8.4 m and height 5.4 m. How much metal sheet, to the nearest m2, is used in making this tank, if `1/15` of the sheet actually used was wasted in making the tank?
The diameter of the cross-section of a water pipe is 5 cm. Water flows through it at 10km/hr into a cistern in the form of a cylinder. If the radius of the base of the cistern is 2.5 m, find the height to which the water will rise in the cistern in 24 minutes.
The total surface area of a cone whose radius is `r/2` and slant height 2l is ______.
The radii of two cylinders are in the ratio of 2:3 and their heights are in the ratio of 5:3. The ratio of their volumes is ______.
If the radius of a cylinder is doubled and height is halved, the volume will be doubled.
Radius of a cylinder is r and the height is h. Find the change in the volume if the height remains same and the radius is halved.
