Advertisements
Advertisements
प्रश्न
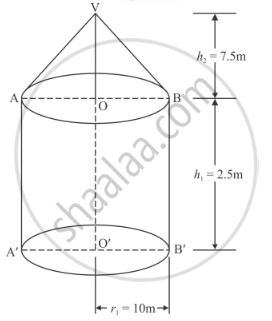
A tent is in the form of a cylinder of diameter 20 m and height 2.5 m, surmounted by a cone of equal base and height 7.5 m. Find the capacity of the tent and the cost of the canvas at Rs 100 per square metre.
उत्तर
Given that:
Radius of the base `r=d/2=20/2=10 m`
Height of the cylinder h1 = 2.5 m
Height of the cone h2 = 7.5 m
Slant height of the cone
`l = sqrt(r^2+h^2)`
`=sqrt(10^2+7.5^2)`
= 12.5 m
The total capacity of the tent is given by
`V=pir^2h_1+1/3pir^2h_2`
`=rxx10^2xx2.5+1/3xxpixx10^2xx7.5`
= π x 250 + π x 250
= 500π m3
The total area of canvas required for the tent is
S = 2πrh + πrl
= 2 x 3.14 x 10 x 2.5 + 3.14 x 10 x 12.5
= π(2 x 10 x 2.5 + 10 x 12.5)
= π(50 + 125)
`= 22/7xx175`
= 550 m2
Therefore, the total cost of the canvas is
= 100 x 550
= Rs. 55000
Hence, the total capacity and cost is V = 500π m3, and Rs. 55000
APPEARS IN
संबंधित प्रश्न
A sphere of diameter 12 cm, is dropped in a right circular cylindrical vessel, partly filled with water. If the sphere is completely submerged in water, the water level in the cylindrical vessel rises by 3 `5/9` cm. Find the diameter of the cylindrical vessel.
A spherical shell of lead, whose external diameter is 18 cm, is melted and recast into a right circular cylinder, whose height is 8 cm and diameter 12 cm. Determine the internal diameter of the shell.
Height of a solid cylinder is 10 cm and diameter 8 cm. Two equal conical hole have been made from its both ends. If the diameter of the holes is 6 cm and height 4 cm, find (i) volume of the cylinder, (ii) volume of one conical hole, (iii) volume of the remaining solid.
The radii of two cylinders are in the ratio 3 : 5 and their heights are in the ratio 2 : 3. What is the ratio of their curved surface areas?
Two cubes have their volumes in the ratio 1 : 27. What is the ratio of their surface areas?
A hemispherical bowl of internal radius 9 cm is full of water. Its contents are emptied into a cylindrical vessel of internal radius 6 cm. Find the height of water in the cylindrical vessel.
A toy is in the form of a cone of radius 3.5 cm mounted on a hemisphere of same radius. The total height of the toy is 15.5 cm. Find the total
surface area of the toy.
A cube whose edge is 20 cm long, has circles on each of its faces painted black. What is the total area of the unpainted surface of the cube if the circles are of the largest possible areas?
What is the area of the largest triangle that can be fitted into a rectangle of length l units and width w units?
The radius of a metal sphere is 3 cm. The sphere is melted and made into a long wire of uniform circular cross-section, whose length is 36 cm. To calculate the radius of wire, complete the following activity.
Radius of the sphere = `square`
Length of the wire = `square`
Let the radius of the wire by r cm.
Now, Volume of the wire = Volume of the `square`
`square` = `square`
r2 × `square` = `square` × `square`
r2 × `square` = `square`
r = `square`
Hence, the radius of the wire is `square` cm.
