Advertisements
Advertisements
प्रश्न
What is the area of the largest triangle that can be fitted into a rectangle of length l units and width w units?
विकल्प
`(lw)/2`
`(lw)/3`
`(lw)/6`
`(lw)/4`
उत्तर
`bb((lw)/2)`
Explanation:
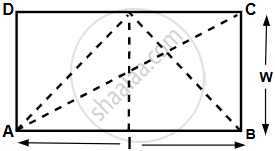
Let ABCD be the rectangle of length l and width w.
Now, we construct a triangle of maximum area inside it in all possible ways.
∵ We know that,
Area of triangle = `1/2` × Base × Height
So, for maximum area, base and height of maximum, length is needed.
Here, maximum base length = `l`
And maximum height = w
∴ Area (maximum) of triangle = `1/2 xx l xx w = (l xx w)/2` sq.units
APPEARS IN
संबंधित प्रश्न
A conical vessel, with base radius 5 cm and height 24 cm, is full of water. This water is emptied into a cylindrical vessel of base radius 10 cm. Find the height to which the water will rise in the cylindrical vessel. (use `pi=22/7`)
A sphere of diameter 12 cm, is dropped in a right circular cylindrical vessel, partly filled with water. If the sphere is completely submerged in water, the water level in the cylindrical vessel rises by 3 `5/9` cm. Find the diameter of the cylindrical vessel.
To fill a swimming pool two pipes are to be used. If the pipe of larger diameter is used for 4 hours and the pipe of smaller diameter for 9 hours, only half the pool can be filled. Find, how long it would take for each pipe to fill the pool separately, if the pipe of smaller diameter takes 10 hours more than the pipe of larger diameter to fill the pool.
A solid metallic sphere of diamter 16 cm is melted and recasted into smaller solid cones, each of radius 4 cm and height 8 cm. Find the number of cones so formed.
A right angled triangle whose sides are 3 cm, 4 cm and 5 cm is revolved about the sides containing the right angle in two days. Find the difference in columes of the two cones so formed. Also, find their curved surfaces.
A hemispherical bowl of internal radius 15 cm contains a liquid. The liquid is to be filled into cylindrical-shaped bottles of diameter 5 cm and height 6 cm. How many bottles are necessary to empty the bowl?
The radii of the ends of a bucket 30 cm high are 21 cm and 7 cm. Find its capacity in litres and the amount of sheet required to make this bucket.
A sphere of maximum volume is cut-out from a solid hemisphere of radius r, what is the ratio of the volume of the hemisphere to that of the cut-out sphere?
The volumes of two cubes are in the ratio 8 : 27. Find the ratio of their surface areas.
Arrange the given objects according to their volume
