Advertisements
Advertisements
प्रश्न
A hemispherical bowl of internal radius 15 cm contains a liquid. The liquid is to be filled into cylindrical-shaped bottles of diameter 5 cm and height 6 cm. How many bottles are necessary to empty the bowl?
उत्तर
Internal radius of hemispherical bowl r = 15 cm
The volume of bowl `= 2/3 pir^3`= volume of liquid
`= 2/3 pi(15)^3`
Volume of liquid = 2250 π cm3
Since, the liquid filled into the cylindrical shaped bottles of radius `5/2 cm`and height 6 cm.
Let n be the no. of bottles.
Therefore,
The volume of liquid = n × volume of cylindrical shaped bottle
`2250pi = n xx pi xx (5/2)^2 xx 6`
`2250 = n xx 25 / 4 xx 6`
`n = (2250 xx 4)/(25 xx 6)`
` n = 60`
Hence, the no. of bottles = 60
APPEARS IN
संबंधित प्रश्न
Sushant has a vessel, of the form of an inverted cone, open at the top, of height 11 cm and radius of top as 2.5 cm and is full of water. Metallic spherical balls each of diameter 0.5 cm are put in the vessel due to which 2/5 th of the water in the vessel flows out. Find how many balls were put in the vessel. Sushant made the arrangement so that the water that flows out irrigates the flower beds. What value has been shown by Sushant?
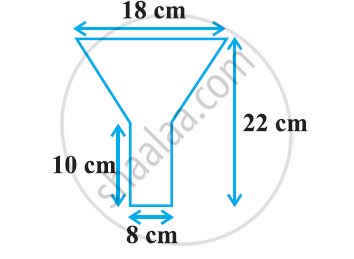
An oil funnel made of tin sheet consists of a 10 cm long cylindrical portion attached to a frustum of a cone. If the total height is 22 cm, diameter of the cylindrical portion is 8 cm and the diameter of the top of the funnel is 18 cm, find the area of the tin sheet required to make the funnel (see the given figure).
A solid sphere of radius 10.5 cm is melted and recast into smaller solid cones, each of radius 3.5 cm and height 3 cm.Find the number of cones so formed.
A hollow sphere of internal and external diameters 4 and 8 cm respectively is melted into a cone of base diameter 8 cm. Find the height of the cone.
A spherical ball of diameter 21 cm is melted and recast into cubes, each of side 1 cm. Find the number of cubes so formed.
Water is flowing at the rate of 6 km/hr through a pipe of diameter 14 cm into a rectangular tank which is 60 m long and 22 m wide. Determine the time in which the level of water in the tank will rise by 7 cm.
If the area of the base of a right circular cone is 3850 cm2 and its height is 84 cm, then find the slant height of the cone.
A sphere of diameter 18 cm is dropped into a cylindrical vessel of diameter 36 cm, partly filled with water. If the sphere is completely submerged, then the water level rises by ______.
The volume of a cube is 2744 cm2. Its surface area is
Assertion (A)
The curved surface area of a cone of base radius 3 cm and height 4 cm is 15π cm2.\
Reason (R)
Volume of a cone = πr2h
- Both Assertion (A) and Reason (R) are true and Reason (R) is a correct explanation of Assertion (A).
- Both Assertion (A) and Reason (R) are true but Reason (R) is not a correct explanation of Assertion (A).
- Assertion (A) is true and Reason (R) is false.
- Assertion (A) is false and Reason (R) is true.
