Advertisements
Advertisements
рдкреНрд░рд╢реНрди
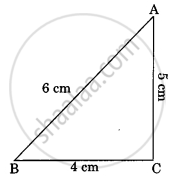
A vertical pole of length 7.5 cm casts a shadow 5 m long on the ground and at the same time a tower casts a shadow 24 m long. Find the height of the tower.
рдЙрддреНрддрд░
Let AB be the vertical stick and BC be its shadow.
Given:
AB = 7.5 m, BC = 5 m
Let PQ be the tower and QR be its shadow.
Given:
QR = 24 m
Let the length of PQ be x m.
In Δ ABC and Δ PQR, we have:
∠ЁЭР┤ЁЭР╡ЁЭР╢ = ∠ЁЭСГЁЭСДЁЭСЕ = 90°
∠ЁЭР┤ЁЭР╢ЁЭР╡ = ∠ЁЭСГЁЭСЕЁЭСД (Angular elevation of the sun at the same time)
Therefore, by A similarity theorem, we get:
Δ ABC ~ Δ PQR
⇒`(AB)/(BC)=(PQ)/(QR)`
⇒ `7.5/5=x/24`
x = ` 7.5/5xx24=36 cm`
Therefore, PQ = 36 m
Hence, the height of the tower is 36 m.
APPEARS IN
рд╕рдВрдмрдВрдзрд┐рдд рдкреНрд░рд╢реНрди
E and F are points on the sides PQ and PR, respectively, of a ΔPQR. For the following case, state whether EF || QR.
PE = 3.9 cm, EQ = 3 cm, PF = 3.6 cm and FR = 2.4 cm
In the given figure, two chords AB and CD of a circle intersect each other at the point P (when produced) outside the circle. Prove that
(i) ΔPAC ∼ ΔPDB
(ii) PA.PB = PC.PD
ABC is a right angled triangle with ∠ABC = 90°. D is any point on AB and DE is perpendicular to AC. Prove that :
- ΔADE ∼ ΔACB.
- If AC = 13 cm, BC = 5 cm and AE = 4 cm. Find DE and AD.
- Find, area of ΔADE : area of quadrilateral BCED.
In ΔABC, ∠ABC = ∠DAC, AB = 8 cm, AC = 4 cm and AD = 5 cm.
- Prove that ΔACD is similar to ΔBCA.
- Find BC and CD.
- Find area of ΔACD : area of ΔABC.
In triangle ABC, DE is parallel to BC; where D and E are the points on AB and AC respectively.
Prove: тИЖADE ~ тИЖABC.
Also, find the length of DE, if AD = 12 cm, BD = 24 cm BC = 8 cm.
In the figure, given below, the medians BD and CE of a triangle ABC meet at G. Prove that:
- ΔEGD ~ ΔCGB and
- BG = 2GD from (i) above.
In the given triangle P, Q and R are the mid-points of sides AB, BC and AC respectively. Prove that triangle PQR is similar to triangle ABC.
Two chords AB and CD of a circle intersect at a point P outside the circle.
Prove that: (i) Δ PAC ~ Δ PDB (ii) PA. PB = PC.PD
O is any point in the interior of ΔABC. Bisectors of ∠AOB, ∠BOC and ∠AOC intersect side AB, side BC, side AC in
F, D and E respectively.
Prove that
BF × AE × CD = AF × CE × BD
A triangle ABC is enlarged, about the point O as centre of enlargement, and the scale factor is 3. Find : A' B', if AB = 4 cm.
A model of an aeroplane is made to a scale of 1 : 400. Calculate : the length, in m, of the aeroplane, if length of its model is 16 cm.
On a map drawn to a scale of 1 : 2,50,000; a triangular plot of land has the following measurements : AB = 3 cm, BC = 4 cm and ∠ABC = 90°.
Calculate : the actual lengths of AB and BC in km.
In ΔABC, point D divides AB in the ratio 5:7, Find: `"AE"/"EC"`
In ΔABC, point D divides AB in the ratio 5:7, Find: BC, If DE = 2.5cm
In ΔABC, DE || BC such that AD =1.5 cm, DB = 3 cm and AE = 1 cm. Find AC.
In a triangle ABC, AB = 4 cm, BC = 4.5 cm and CA = 5 cm. Construct ΔABC. Find the image A'B'C of the ΔABC obtained by enlarging it by a scale factor 2. Measure the sides of the image A'B'C' and show that AB:A'B' = AC:B'C' = CA:C'A'
In the adjacent figure, тИЖACB ~ тИЖAPQ. If BC = 8 cm, PQ = 4 cm, BA = 6.5 cm and AP = 2.8 cm, find CA and AQ
If figure OPRQ is a square and ∠MLN = 90°. Prove that тИЖLOP ~ тИЖRPN
If figure OPRQ is a square and ∠MLN = 90°. Prove that QR2 = MQ × RN
If BD ⊥ AC and CE ⊥ AB, prove that `"CA"/"AB" = "CE"/"DB"`
Are triangles in figure similar? If yes, then write the test of similarity.
If ΔABC ~ ΔLMN and ∠B = 40°, then ∠M = ? Give reason.
In fig. BP ⊥ AC, CQ ⊥ AB, A−P−C, and A−Q−B then show that ΔAPB and ΔAQC are similar.
In ΔAPB and ΔAQC
∠APB = [ ]° ......(i)
∠AQC = [ ]° ......(ii)
∠APB ≅ ∠AQC .....[From (i) and (ii)]
∠PAB ≅ ∠QAC .....[______]
ΔAPB ~ ΔAQC .....[______]
There are two poles having heights 8 m and 4 m on plane ground as shown in fig. Because of sunlight shadows of smaller pole is 6m long, then find the length of shadow of longer pole.
Two triangles are similar. Smaller triangle’s sides are 4 cm, 5 cm, 6 cm. Perimeter of larger triangle is 90 cm then find the sides of larger triangle.
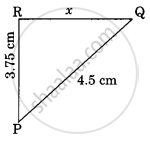
In the given figure ΔABC ~ ΔPQR. The value of x is
 |
 |
In figure, if AD = 6cm, DB = 9cm, AE = 8cm and EC = 12cm and ∠ADE = 48°. Find ∠ABC.
In the adjoining diagram the length of PR is ______.
