Advertisements
Advertisements
प्रश्न
In the figure, given below, the medians BD and CE of a triangle ABC meet at G. Prove that:

- ΔEGD ~ ΔCGB and
- BG = 2GD from (i) above.
उत्तर
i. Since, BD and CE are medians
AD = DC
AE = BE
Hence, by converse of Basic Proportionality theorem,
ED || BC
In ΔEGD and ΔCGB,
∠DEG = ∠GCB ...(Alternate angles)
∠EGD = ∠BGC ...(Vertically opposite angles)
ΔEGD ~ ΔCGB ...(AA similarity)
ii. Since, ΔEGD ~ ΔCGB
`(GD)/(GB) = (ED)/(BC)` ...(1)
In ΔAED and ΔABC,
∠AED = ∠ABC ...(Corresponding angles)
∠EAD = ∠BAC ...(Common)
∴ ΔEAD ∼ ΔBAC ...(AA similarity)
∴ `(ED)/(BC) = (AE)/(AB) = 1/2 ` ...(Since, E is the mid-point of AB)
`=> (ED)/(BC) = 1/2`
From (1),
`(GD)/(GB) = 1/2`
GB = 2GD
APPEARS IN
संबंधित प्रश्न
In figure, ∠CAB = 90º and AD ⊥ BC. If AC = 75 cm, AB = 1 m and BD = 1.25 m, find AD.
In the given figure, AB || EF || DC; AB = 67.5 cm, DC = 40.5 cm and AE = 52.5 cm.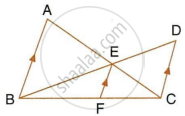
- Name the three pairs of similar triangles.
- Find the lengths of EC and EF.
P and Q are points on the sides AB and AC respectively of a ΔABC. If AP = 2cm, PB = 4cm, AQ = 3cm and QC = 6cm, show that BC = 3PQ.
The areas of two similar triangles are `64cm^2` and `100cm^2` respectively. If a median of the smaller triangle is 5.6cm, find the corresponding median of the other.
If ΔABC ~ ΔDEF, then writes the corresponding congruent angles and also write the ratio of corresponding sides.
AM and DN are the altitudes of two similar triangles ABC and DEF. Prove that: AM : DN = AB : DE.
Given is a triangle with sides 3 cm, 5 cm and 6 cm. Find the sides of a triangle which is similar to the given triangle and its shortest side is 4.5 cm.
In ΔABC, AB = 8cm, AC = 10cm and ∠B = 90°. P and Q are the points on the sides AB and AC respectively such that PQ = 3cm ad ∠PQA = 90. Find: The area of ΔAQP.
Areas of two similar triangles are 225 cm2 and 81 cm2. If side of smaller triangle is 12 cm, find corresponding side of major triangle.
In the given figure, ∠ACB = ∠CDA, AC = 8cm, AD = 3cm, then BD is ______.

