Advertisements
Advertisements
प्रश्न
In ΔABC, AB = 8cm, AC = 10cm and ∠B = 90°. P and Q are the points on the sides AB and AC respectively such that PQ = 3cm ad ∠PQA = 90. Find: The area of ΔAQP.
उत्तर
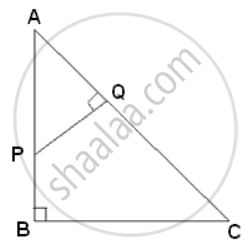
In ΔAQP and ΔABC
∠A = ∠A
∠PQA = ∠ABC ...(right angles)
Therefore, ΔAQP ∼ ΔABC
By Pythagoras theorem,
BC2 = AC2 - AB2
⇒ BC2 = 102 - 82
⇒ BC2 = 100 - 64
⇒ BC2 = 36
⇒ BC = 6cm
Area (ΔABC) `(1)/(2) xx "AB" xx "BC"`
Area (ΔABC) `(1)/(2) xx 8 xx 6`
Area (ΔABC) = 24cm2
Since ΔAQP ∼ ΔABC
`("Area"(Δ"AQP"))/("Area"(Δ"ABC")) = "PQ"^2/"BC"^2`
`("Area"(Δ"AQP"))/("Area"(Δ"ABC")) = 3^2/6^2`
⇒ Area(ΔAQP) = `(9 xx 24)/(36)`
⇒ Area(ΔAQP) = 6cm2 .
APPEARS IN
संबंधित प्रश्न
In the adjoining figure, ABC is a right angled triangle with ∠BAC = 90°.
1) Prove ΔADB ~ ΔCDA.
2) If BD = 18 cm CD = 8 cm Find AD.
3) Find the ratio of the area of ΔADB is to an area of ΔCDA.

In the given figure, ABC is a triangle. DE is parallel to BC and `(AD)/(DB) = 3/2`.
- Determine the ratios `(AD)/(AB)` and `(DE)/(BC)`.
- Prove that ∆DEF is similar to ∆CBF. Hence, find `(EF)/(FB).`
- What is the ratio of the areas of ∆DEF and ∆BFC?
In the given figure, ΔOAB ~ ΔOCD. If AB = 8cm, BO = 6.4cm, OC = 3.5cm and CD = 5cm, find (i) OA (ii) DO.
The areas of two similar triangles are `64cm^2` and `100cm^2` respectively. If a median of the smaller triangle is 5.6cm, find the corresponding median of the other.
The dimensions of the model of a multistorey building are 1.2 m × 75 cm × 2 m. If the scale factor is 1 : 30; find the actual dimensions of the building.
In the figure, given below, PQR is a right-angle triangle right angled at Q. XY is parallel to QR, PQ = 6 cm, PY = 4 cm and PX : XQ = 1 : 2. Calculate the lengths of PR and QR.

If ΔABC, D and E are points on AB and AC. Show that DE || BC for each of the following case or not:
AD = 5.7cm, BD = 9.5cm, AE = 3.3cm, and EC = 5.5cm
In a right-angled triangle ABC, ∠B = 90°, P and Q are the points on the sides AB and AC such as PQBC, AB = 8 cm, AQ = 6 cm and PA:AB = 1:3. Find the lengths of AC and BC.
D and E are points on the sides AB and AC of ΔABC such that DE | | BC and divides ΔABC into two parts, equal in area. Find `"BD"/"AB"`.
If figure OPRQ is a square and ∠MLN = 90°. Prove that ∆LOP ~ ∆RPN

