Advertisements
Advertisements
प्रश्न
ABCD is a quadrilateral P, Q, R and S are the mid-points of AB, BC, CD and AD. Prove that PQRS is a parallelogram.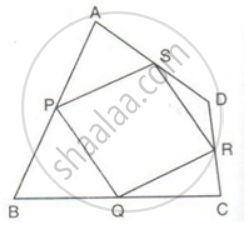
उत्तर
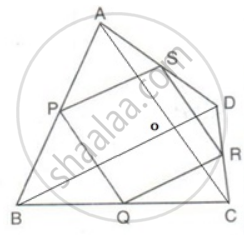
Join AC and BD
In ΔABC,
P and Q are mid-point of AB and BC respectively.
Therefore, PQ || AC and PQ = `(1)/(2)"AC"` ........(i)
In ΔADC,
S and R are mid-point of AD and DC respectively.
Therefore, SR || AC and SR = `(1)/(2)"AC"` ........(ii)
From (i) and (ii)
PQ || SR and PQ = SR
Therefore, PQRS is a parallelogram.
APPEARS IN
संबंधित प्रश्न
PQRS is a parallelogram. PQ is produced to T so that PQ = QT. Prove that PQ = QT. Prove that ST bisects QR.
P is a point on side KN of a parallelogram KLMN such that KP : PN is 1 : 2. Q is a point on side LM such that LQ : MQ is 2 : 1. Prove that KQMP is a parallelogram.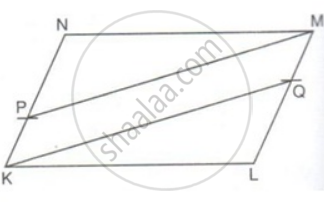
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
PMRN is a parallelogram.
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
MN bisects QS.
In the given figure, PQRS is a trapezium in which PQ ‖ SR and PS = QR. Prove that: ∠PSR = ∠QRS and ∠SPQ = ∠RQP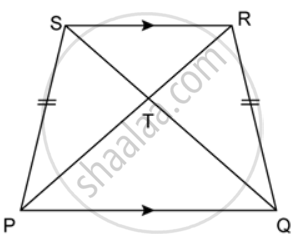
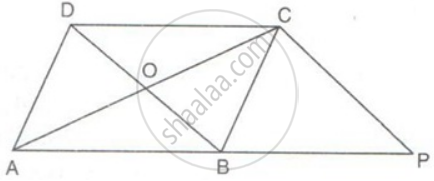
Prove that the diagonals of a parallelogram divide it into four triangles of equal area.
The diagonals AC and BC of a quadrilateral ABCD intersect at O. Prove that if BO = OD, then areas of ΔABC an ΔADC area equal.
In the given figure, AB ∥ SQ ∥ DC and AD ∥ PR ∥ BC. If the area of quadrilateral ABCD is 24 square units, find the area of quadrilateral PQRS.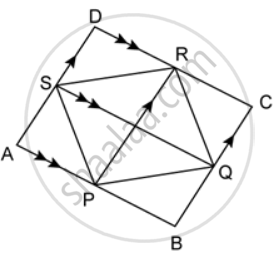
In ΔABC, the mid-points of AB, BC and AC are P, Q and R respectively. Prove that BQRP is a parallelogram and that its area is half of ΔABC.
In the figure, ABCD is a parallelogram and CP is parallel to DB. Prove that: Area of OBPC = `(3)/(4)"area of ABCD"`