Advertisements
Advertisements
प्रश्न
Prove that the diagonals of a parallelogram divide it into four triangles of equal area.
उत्तर

The diagonals of a parallelogram bisect each other.
Therefore, O is the mid-point of AC and BD.
Bo is the median in ΔABC.
Therefore, it will divide it into two triangles of equal areas
∴ ar(ΔAOB) = ar(ΔBOC)......(i)
In ΔBCD, CO is the median.
∴ ar(ΔBOC) = ar(ΔCOD).......(ii)
Similarly, ar(ΔCOD) = ar(ΔAOD)......(iii)
From (i), (ii) and (iii)
ar(ΔAOB) = ar(ΔBOC) = ar(ΔCOD) = ar(ΔAOD)
Hence, diagonals of a parallelogram divide it into foor triangles of equal areas.
APPEARS IN
संबंधित प्रश्न
ABCD is a rectangle with ∠ADB = 55°, calculate ∠ABD.
Prove that if the diagonals of a parallelogram are equal then it is a rectangle.
ABCD is a quadrilateral P, Q, R and S are the mid-points of AB, BC, CD and AD. Prove that PQRS is a parallelogram.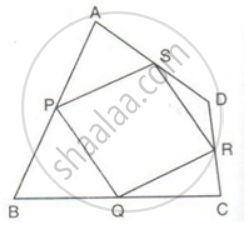
Prove that the line segment joining the mid-points of the diagonals of a trapezium is parallel to each of the parallel sides, and is equal to half the difference of these sides.
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
MN bisects QS.
ABCD is a trapezium in which side AB is parallel to side DC. P is the mid-point of side AD. IF Q is a point on the Side BC such that the segment PQ is parallel to DC, prove that PQ = `(1)/(2)("AB" + "DC")`.
In the given figure, PQRS is a parallelogram in which PA = AB = Prove that: SA ‖ QB and SA = QB.
In the given figure, PQRS is a parallelogram in which PA = AB = Prove that: SAQB is a parallelogram.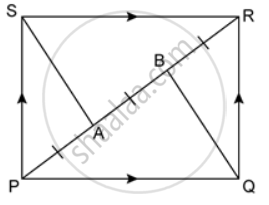
PQRS is a parallelogram and O is any point in its interior. Prove that: area(ΔPOQ) + area(ΔROS) - area(ΔQOR) + area(ΔSOP) = `(1)/(2)`area(|| gm PQRS)
The medians QM and RN of ΔPQR intersect at O. Prove that: area of ΔROQ = area of quadrilateral PMON.
