Advertisements
Advertisements
प्रश्न
ABCD is a rhombus whose diagonals intersect at O. If AB = 10 cm, diagonal BD = 16 cm, find the length of diagonal AC.
Advertisements
उत्तर
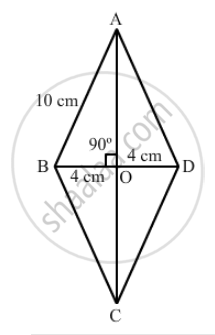
\[\text{ We know that the diagonals of a rhombus bisect each other at right angles }. \]
\[ \therefore BO = \frac{1}{2}BD = (\frac{1}{2} \times 16) cm\]
\[ = 8cm\]
\[AB = 10 \text{ cm and }\angle AOB = 90°\]
\[\text{ From right } ∆ OAB: \]
\[ {AB}^2 = {AO}^2 + {BO}^2 \]
\[ \Rightarrow {AO}^2 = ( {AB}^2 -{BO}^2 )\]
\[ \Rightarrow {AO}^2 = (10 )^2 - (8 )^2 {cm}^2 \]
\[ \Rightarrow {AO}^2 = (100 - 64) {cm}^2 = 36 {cm}^2 \]
\[ \Rightarrow AO = \sqrt{36} cm = 6cm\]
\[ \therefore AC = 2 \times AO = (2 \times 6) cm = 12 cm\]
APPEARS IN
संबंधित प्रश्न
Name the quadrilaterals whose diagonals are perpendicular bisectors of each other
Can the following figure be parallelogram. Justify your answer.
In the following figure RISK and CLUE are parallelograms. Find the measure of x.
Diagonals of parallelogram ABCD intersect at O as shown in the following fegure. XY contains O, and X, Y are points on opposite sides of the parallelogram. Give reasons for each of the following:
(i) OB = OD
(ii) ∠OBY = ∠ODX
(iii) ∠BOY = ∠DOX
(iv) ∆BOY ≅ ∆DOX
Now, state if XY is bisected at O.
In the following Figure ABCD is a arallelogram, CE bisects ∠C and AF bisects ∠A. In each of the following, if the statement is true, give a reason for the same:
(i) ∠A = ∠C
(ii) \[\angle FAB = \frac{1}{2}\angle A\]
(iii) \[\angle DCE = \frac{1}{2}\angle C\]
(iv) \[\angle CEB = \angle FAB\]
(v) CE || AF
Which of the following statement is true for a rhombus?
It is a quadrilateral.
Which of the following statement is true for a rhombus?
It can be a square.
Measure of one angle of a rhombus is 50°, find the measures of remaining three angles.
If all sides of a quadrilateral are equal, it is a ______.
Diagonals of a quadrilateral are perpendicular to each other. Is such a quadrilateral always a rhombus? Give a figure to justify your answer.
