Advertisements
Advertisements
प्रश्न
Answer the following question.
Discuss the following as special cases of elastic collisions and obtain their exact or approximate final velocities in terms of their initial velocities.
- Colliding bodies are identical.
- A very heavy object collides on a lighter object, initially at rest.
- A very light object collides on a comparatively much massive object, initially at rest.
उत्तर
The final velocities after a head-on elastic collision is given as,
`"v"_1 = "u"_1 [("m"_1 - "m"_2)/("m"_1 + "m"_2)] + "u"_2["2m"_2/("m"_1 + "m"_2)]`
`"v"_1 = "u"_1["2m"_1/("m"_1 + "m"_2)] + "u"_2[("m"_2 - "m"_1)/("m"_1 + "m"_2)]`
- Colliding bodies are identical
If m1 = m2, then v1 = u2 and v2 = u1. Thus, objects will exchange their velocities after head on elastic collision. - A very heavy object collides with a lighter object, initially at rest.
Let m1 be the mass of the heavier body and m2 be the mass of the lighter body i.e., m1 >> m2; the lighter particle is at rest i.e., u2 = 0 then,
m1 ± m2 ≅ m1 and `"m"_2/("m"_1 + "m"_2) ~= 0,`
∴ v1 ≅ u1 and v2 ≅ 2u1
i.e., the heavier colliding body is left unaffected and the lighter body which is struck travels with double the speed of the massive striking body. - A very light object collides on a comparatively much massive object, initially at rest.
If m1 is the mass of a light body and m2 is the mass of a heavy body i.e., m1 << m2 and u2 = 0. Thus, m1 can be neglected.
Hence v1 ≅ - u1 and v2 ≅ 0.
i.e., the tiny (lighter) object rebounds with the same speed while the massive object is unaffected.
APPEARS IN
संबंधित प्रश्न
State if the following statement is true or false. Give a reason for your answer.
In an inelastic collision, the final kinetic energy is always less than the initial kinetic energy of the system.
Answer carefully, with reason:
In an inelastic collision of two billiard balls, is the total kinetic energy conserved during the short time of collision of the balls (i.e., when they are in contact)?
A molecule in a gas container hits a horizontal wall with speed 200 m s–1 and angle 30° with the normal, and rebounds with the same speed. Is momentum conserved in the collision? Is the collision elastic or inelastic?
Arrive at an expression for elastic collision in one dimension and discuss various cases.
A bomb of mass 9 kg explodes into two pieces of mass 3 kg and 6 kg. The velocity of mass 3 kg is 16 m/s, The kinetic energy of mass 6 kg is ____________.
A mass M moving with velocity 'v' along x-axis collides and sticks to another mass 2M which is moving along Y-axis with velocity 3v. After collision, the velocity of the combination is ______.
A particle of mass 'm' collides with another stationary particle of mass 'M'. A particle of mass 'm' stops just after collision. The coefficient of restitution is ______.
A wooden block of mass 'M' moves with velocity 'v ' and collides with another block of mass '4M' which is at rest. After collision, the block of mass 'M' comes to rest. The coefficient of restitution will be ______.
Two bodies of masses 3 kg and 2 kg collide bead-on. Their relative velocities before and after collision are 20 m/s and 5 m/s respectively. The loss of kinetic energy of the system is ______.
A smooth sphere of mass 'M' moving with velocity 'u' directly collides elastically with another sphere of mass 'm' at rest. After collision, their final velocities are V' and V respectively. The value of V is given by ______.
During inelastic collision between two bodies, which of the following quantities always remain conserved?
A cricket ball of mass 150 g moving with a speed of 126 km/h hits at the middle of the bat, held firmly at its position by the batsman. The ball moves straight back to the bowler after hitting the bat. Assuming that collision between ball and bat is completely elastic and the two remain in contact for 0.001s, the force that the batsman had to apply to hold the bat firmly at its place would be ______.
Two blocks M1 and M2 having equal mass are free to move on a horizontal frictionless surface. M2 is attached to a massless spring as shown in figure. Iniially M2 is at rest and M1 is moving toward M2 with speed v and collides head-on with M2.
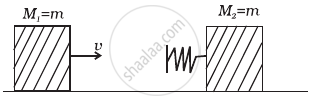
- While spring is fully compressed all the KE of M1 is stored as PE of spring.
- While spring is fully compressed the system momentum is not conserved, though final momentum is equal to initial momentum.
- If spring is massless, the final state of the M1 is state of rest.
- If the surface on which blocks are moving has friction, then collision cannot be elastic.
In an elastic collision of two billiard balls, which of the following quantities remain conserved during the short time of collision of the balls (i.e., when they are in contact).
- Kinetic energy.
- Total linear momentum?
Give reason for your answer in each case.
The bob A of a pendulum released from horizontal to the vertical hits another bob B of the same mass at rest on a table as shown in figure.
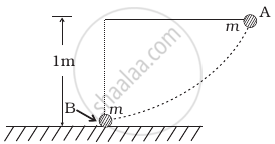
If the length of the pendulum is 1 m, calculate
- the height to which bob A will rise after collision.
- the speed with which bob B starts moving. Neglect the size of the bobs and assume the collision to be elastic.
A rod of mass M and length L is lying on a horizontal frictionless surface. A particle of mass 'm' travelling along the surface hits at one end of the rod with velocity 'u' in a direction perpendicular to the rod. The collision is completely elastic. After collision, particle comes to rest. The ratio of masses `(m/M)` is `1/x`. The value of 'x' will be ______.
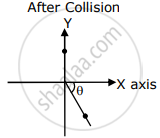
A ball of mass 10 kg moving with a velocity of 10`sqrt3` ms–1 along the X-axis, hits another ball of mass 20 kg which is at rest. After collision, the first ball comes to rest and the second one disintegrates into two equal pieces. One of the pieces starts moving along Y-axis at a speed of 10 m/s. The second piece starts moving at a speed of 20 m/s at an angle θ (degree) with respect to the X-axis.
The configuration of pieces after the collision is shown in the figure.
The value of θ to the nearest integer is ______.
A particle of mass m with an initial velocity u`hat"i"` collides perfectly elastically with a mass 3m at rest. It moves with a velocity v`hat"j"` after collision, then, v is given by :
A ball falls from a height of 1 m on a ground and it loses half its kinetic energy when it hits the ground. What would be the total distance covered by the ball after sufficiently long time?
An insect moves with a constant velocity v from one corner of a room to other corner which is opposite of the first corner along the largest diagonal of room. If the insect can not fly and dimensions of room is a × a × a, then the minimum time in which the insect can move is `"a"/"v"`. times the square root of a number n, then n is equal to ______.
A bag of sand of mass 9.8 kg is suspended by a rope. A bullet of 200 g travelling with speed 10 ms-1 gets embedded in it, then loss of kinetic energy will be ______.
An alpha-particle of mass m suffers 1-dimensional elastic collision with a nucleus at rest of unknown mass. It is scattered directly backwards losing, 64% of its initial kinetic energy. The mass of the nucleus is ______.
A sphere of mass 'm' moving with velocity 'v' collides head-on another sphere of same mass which is at rest. The ratio of final velocity of second sphere to the initial velocity of the first sphere is ______. ( e is coefficient of restitution and collision is inelastic)
Three identical blocks A, B and C are placed on horizontal frictionless surface. The blocks A and C are at rest. But A is approaching towards B with a speed 10 m/s. The coefficient of restitution for all collision is 0.5. The speed of the block C just after the collision is ______.

Answer carefully, with reason:
Is the total linear momentum conserved during the short time of an inelastic collision of two balls ?
