Advertisements
Advertisements
प्रश्न
Answer the following question.
Show that the centripetal force on a particle undergoing uniform circular motion is -mrω2.
उत्तर
- Suppose a particle is performing U.C.M in anticlockwise direction. The co-ordinate axes are chosen as shown in the figure.
Let, A = initial position of the particle which lies on positive X-axis
P = instantaneous position after time t
θ = angle made by radius vector
ω = constant angular speed
`vec"r"` = instantaneous position vector at time t - From the figure,
`vec"r" = hat"i""x" + hat"j""y"`
where, `hat"i" and hat"j"` are unit vectors along X-axis and Y-axis respectively.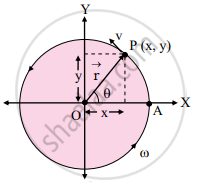
- Also, x = r cos θ and y = r sin θ
∴ `vec"r" = ["r"hat"i"cos theta + "r"hat"j"sin theta]`
But θ = ωt
∴ `vec"r" = ["r"hat"i" cos omega"t" + "r"hat"j" sin omega"t"]` ....(1) - Velocity of the particle is given as rate of change of position vector.
∴ `vec"v" = (vec"dr")/"dt" = "d"/"dt" ["r"hat"i" cos omega"t" + "r" hat"j" sin omega"t"]`
`= "r"["d"/"dt" cos omega"t"]hat"i" + "r"["d"/"dt" sin omega"t"]hat"j"`
∴ `vec"v" = - "r"omega hat"i" sin omega"t" + "r"omega hat"j" cos omega"t"`
∴ `vec"v" = "r"omega (-hat"i" sin omega"t" + hat"j" cos omega"t")` - Further, instantaneous linear acceleration of the particle at instant t is given by,
`vec"a" = (vec"dv")/"dt" = "d"/"dt"["r"omega(- hat"i" sin omega "t" + hat"j" cos omega"t")]`
`= "r"omega ["d"/"dt" (- hat"i" sin omega"t" + hat"j" cos omega "t")]`
`= "r"omega ["d"/"dt" (- sin omega"t")hat"i" + "d"/"dt"(cos omega "t")hat"j"]`
`= "r"omega(-omega hat"i" cos omega"t" - omega hat"j" sin omega "t")`
`= - "r"omega^2(hat"i" cos omega "t" + hat"j" sin omega"t")`
∴ `vec"a" = - omega^2("r"hat"i" cos omega"t" + "r" hat"j" sin omega "t")` ....(2) - From equation (1) and (2),
`vec"a" = - omega^2vec"r"` ....(3)
The negative sign shows that the direction of acceleration is opposite to the direction of the position vector. Equation (3) is the centripetal acceleration. - The magnitude of centripetal acceleration is given by,
a = `omega^2"r"` - The force providing this acceleration should also be in the same direction, hence centripetal.
∴ `vec"F" = "m"vec"a" = - "m"omega^2vec"r"`
This is the expression for the centripetal force on a particle undergoing uniform circular motion. - Magnitude of F = `"m"omega^2"r" = "mv"^2/"r"` = mωv
APPEARS IN
संबंधित प्रश्न
For a particle performing uniform circular motion `vecv=vecomegaxxvecr`obtain an expression for linear acceleration of the particle performing non-uniform circular motion.
Read the statement below carefully and state, with reason, if it is true or false:
The acceleration vector of a particle in uniform circular motion averaged over one cycle is a null vector.
A cyclist is riding with a speed of 27 km/h. As he approaches a circular turn on the road of radius 80 m, he applies brakes and reduces his speed at the constant rate of 0.50 m/s every second. What is the magnitude and direction of the net acceleration of the cyclist on the circular turn?
A vehicle is moving on a circular track whose surface is inclined towards the horizon at an angle of 10°. The maximum velocity with which it can move safely is 36 km / hr. Calculate the length of the circular track. [π = 3.142]
Why is the motion of a body moving with a constant speed around a circular path said to be accelerated?
Earth moves around the sun with uniform velocity.
Explain the meaning of uniform circular motion. Give one example of such motion.
Differentiate between uniform linear motion and uniform circular motion.
State True or False
The earth moves around the sun with a uniform.
In a uniform circular motion, the speed continuously changes because of the direction of motion changes.
Define Uniform circular motion.
Solve the following problem.
A projectile is thrown at an angle of 30° to the horizontal. What should be the range of initial velocity (u) so that its range will be between 40m and 50 m? Assume g = 10 m s-2.
The angle subtended by the vector A = `5hat"i" + 3hat"j" + 12hat"k"` with the X-axis is ______.
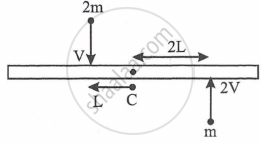
A uniform rod of length '6L' and mass '8 m' is pivoted at its centre 'C'. Two masses 'm' and ' 2m' with speed 2v, v as shown strikes the rod and stick to the rod. Initially the rod is at rest. Due to impact, if it rotates with angular velocity 'w1' then 'w' will be ________.
The ratio of the angular speed of minute hand and hour hand of a watch is ____________.
A small sphere is attached to a cord and rotates in a vertical circle about a point O. If the average speed of the sphere is increased, the cord is most likely to break at the orientation when the mass is at ____________.
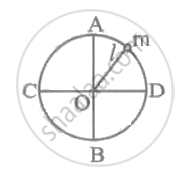
Select the WRONG statement.
Two particles P and Q are moving in concentric circles of rarui rp and rQ respectively. If their period of revolutions are in ratio 2 : 3, then ratio of their centripetal acceleration is ____________.
Statement A: Uniform circular motion is a case of accelerated motion
Statement B: In the third equation of motion we do not have the term time
The motion of the bus is ______ motion.
Earth can be thought of as a sphere of radius 6400 km. Any object (or a person) is performing circular motion around the axis of earth due to earth’s rotation (period 1 day). What is acceleration of object on the surface of the earth (at equator) towards its centre? what is it at latitude θ? How does these accelerations compare with g = 9.8 m/s2?
Earth also moves in circular orbit around sun once every year with on orbital radius of 1.5 × 1011 m. What is the acceleration of earth (or any object on the surface of the earth) towards the centre of the sun? How does this acceleration compare with g = 9.8 m/s2?
A wet open umbrella is held upright and is rotated about the handle at a uniform rate of 21 revolutions in 44 s. If the rim of the umbrella is a circle of 1 metre in diameter and the height of the rim above the floor is 1.5 m, the drops of water spun off the rim and hit the floor at a horizontal ______ m from the umbrella.
A wheel is rotating at 900 rpm about its axis. When the power is cut off it comes to rest in 1 min. The angular retardation (assumed to be uniform (in rad s-1) is ______.
A thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω. Another disc of same dimensions, but of mass `1/4`M is placed gently on the first disc co-axially. The. angular velocity of the system is ______.
A wheel is Subjected to uniform angular acceleration about its axis. Initially. its angular velocity is zero. In the first 2 s, it rotates through an angle θ1, in the next 2 s, it rotates through an angle θ2. The ratio of `theta_2/theta_1` is ______.
A body of mass m is moving in circle of radius r with a constant speed v. The work done by the centripetal force in moving the body over half the circumference of the circle is ______.
The kinetic energy K of a particle moving along a circle of radius R depends on the distance covered s as K = as2, where a is a constant. The force acting on the particle is ______.
