Advertisements
Advertisements
प्रश्न
Answer the following question.
What is thermal stress?
उत्तर
- Consider a metallic rod of length l0 fixed between two rigid supports at T °C.
- If the temperature of rod is increased by ΔT, length of the rod would become, l = l0 (1 + αΔT) Where, α is the coefficient of linear expansion of the material of the rod.
- But the supports prevent the expansion of the rod. As a result, rod exerts stress on the supports. Such stress is termed as thermal stress.
APPEARS IN
संबंधित प्रश्न
If two bodies are in thermal equilibrium in one frame, will they be in thermal equilibrium in all frames?
If an automobile engine is overheated, it is cooled by pouring water on it. It is advised that the water should be poured slowly with the engine running. Explain the reason.
A system X is neither in thermal equilibrium with Y nor with Z. The systems Y and Z
A gas thermometer measures the temperature from the variation of pressure of a sample of gas. If the pressure measured at the melting point of lead is 2.20 times the pressure measured at the triple point of water, find the melting point of lead.
A steel rod is clamped at its two ends and rests on a fixed horizontal base. The rod is unstrained at 20°C.
Find the longitudinal strain developed in the rod if the temperature rises to 50°C. Coefficient of linear expansion of steel = 1.2 × 10–5 °C–1.
Answer the following question.
State applications of thermal expansion.
A glass flask has a volume 1 × 10−4 m3. It is filled with a liquid at 30°C. If the temperature of the system is raised to 100°C, how much of the liquid will overflow? (Coefficient of volume expansion of glass is 1.2 × 10−5 (°C)−1 while that of the liquid is 75 × 10−5 (°C)−1).
Solve the following problem.
In olden days, while laying the rails for trains, small gaps used to be left between the rail sections to allow for thermal expansion. Suppose the rails are laid at room temperature 27 °C. If maximum temperature in the region is 45 °C and the length of each rail section is 10 m, what should be the gap left given that α = 1.2 × 10–5K–1 for the material of the rail section?
Solve the following problem.
A blacksmith fixes iron ring on the rim of the wooden wheel of a bullock cart. The diameter of the wooden rim and the iron ring are 1.5 m and 1.47 m respectively at room temperature of 27 °C. To what temperature the iron ring should be heated so that it can fit the rim of the wheel? (αiron = 1.2 × 10–5K–1).
An iron plate has a circular hole of a diameter 11 cm. Find the diameter of the hole when the plate is uniformly heated from 10° C to 90° C.`[alpha = 12 xx 10^-6//°"C"]`
A metal rod is heated to t°C. A metal rod has length, area of cross-section, Young's modulus and coefficient of linear expansion as 'L', 'A', 'Y' and 'a' respectively. When the rod is heated, the work performed is ______.
A bimetallic strip is made of aluminium and steel (αAl > αsteel) . On heating, the strip will ______.
An aluminium sphere is dipped into water. Which of the following is true?
As the temperature is increased, the time period of a pendulum ______.
The radius of a metal sphere at room temperature T is R, and the coefficient of linear expansion of the metal is α. The sphere is heated a little by a temperature ∆T so that its new temperature is T + ∆T. The increase in the volume of the sphere is approximately ______.
A student records the initial length l, change in temperature ∆T and change in length ∆l of a rod as follows:
| S.No. | l(m) | ∆T (C) | ∆l (m) |
| 1. | 2 | 10 | `4 xx 10^-4` |
| 2. | 1 | 10 | `4 xx 10^-4` |
| 3. | 2 | 20 | `2 xx 10^-4` |
| 4. | 3 | 10 | `6 xx 10^-4` |
If the first observation is correct, what can you say about observations 2, 3 and 4.
Calculate the stress developed inside a tooth cavity filled with copper when hot tea at temperature of 57°C is drunk. You can take body (tooth) temperature to be 37°C and α = 1.7 × 10–5/°C, bulk modulus for copper = 140 × 109 N/m2.
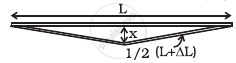
A rail track made of steel having length 10 m is clamped on a raillway line at its two ends (figure). On a summer day due to rise in temperature by 20° C, it is deformed as shown in figure. Find x (displacement of the centre) if αsteel = 1.2 × 10–5/°C.
At what temperature a gold ring of diameter 6.230 cm be heated so that it can be fitted on a wooden bangle of diameter 6.241 cm? Both diameters have been measured at room temperature (27°C). (Given: coefficient of linear thermal expansion of gold αL = 1.4 × 10-5 K-1).
Each side of a box made of metal sheet in cubic shape is 'a' at room temperature 'T', the coefficient of linear expansion of the metal sheet is 'α'. The metal sheet is heated uniformly, by a small temperature ΔT, so that its new temeprature is T + ΔT. Calculate the increase in the volume of the metal box.
A metal ball immersed in water weighs w1 at 0°C and w2 at 50°C. The coefficient of cubical expansion of metal is less than that of water. Then ______.
A disc is rotating freely about its axis. The percentage change in angular velocity of a disc if temperature decreases by 20°C is ______.
(coefficient of linear expansion of material of disc is 5 × 10-4/°C)
A glass flask is filled up to a mark with 50 cc of mercury at 18°C. If the flask and contents are heated to 38°C, how much mercury will be above the mark? (α for glass is 9 × 10-6/°C and coefficient of real expansion of mercury is 180 × 10-6/°C)
