Advertisements
Advertisements
प्रश्न
Consider a small cube of volume 1 mm3 at the centre of a circular loop of radius 10 cm carrying a current of 4 A. Find the magnetic energy stored inside the cube.
उत्तर
Given:-
Current in the loop, i = 4 A
Radius of the loop, r = 10 cm = 0.1 m
Volume of the cube, V = 1 mm3 = 1 × 10-9m
Magnetic field intensity at the centre of the circular loop:-
\[B = \frac{\mu_0 i}{2r}\]
\[ = \frac{(4\pi \times {10}^{- 7} ) \times 4}{2 \times 0 . 1}\]
\[ = 8\pi \times {10}^{- 6} T\]
Magnetic energy density = \[\frac{B^2}{2 \mu_0}\]
otal energy stored in volume V:-
\[U=\frac{B^2 V}{2 \mu_0}\]
\[= \frac{(8\pi \times {10}^{- 6} )^2 \times (1 \times {10}^{- 9} )}{(4\pi \times {10}^{- 7} ) \times 2}\]
\[ = 8\pi \times {10}^{- 14} J\]
APPEARS IN
संबंधित प्रश्न
Define the coefficient of self-induction.
Write the SI unit and dimention of of co-efficient of self induction
The currents flowing in the two coils of self-inductance L1 = 16 mH and L2 = 12 mH are increasing at the same rate. If the power supplied to the two coil is equal, find the ratio of the currents ?
The currents flowing in the two coils of self-inductance L1 = 16 mH and L2 = 12 mH are increasing at the same rate. If the power supplied to the two coil is equal, find the ratio of the energies stored in the two coils at a given instant ?
An average emf of 20 V is induced in an inductor when the current in it is changed from 2.5 A in one direction to the same value in the opposite direction in 0.1 s. Find the self-inductance of the inductor.
Explain why the inductance of two coils connected in parallel is less than the inductance of either coil.
Two pure inductors each of self-inductance L are connected in series, the net inductance is ______
Obtain an expression for the self-inductance of a solenoid.
Two rods of same material and volume having circular cross-section are subjected to tension T. Within the elastic limit, same force is applied to both the rods. Diameter of the first rod is half of the second rod, then the extensions of first rod to second rod will be in the ratio
When the number of turns in a coil is tripled without any change in the length of the coil, its self-inductance ______
The coefficient of self-inductance of a solenoid is 0.20 mH. If a core of soft iron of relative permeability 900 is inserted, then the coefficient of self-inductance will become nearly ______
A toroidal solenoid with air core has an average radius 'R', number of turns 'N' and area of cross-section 'A'. The self-inductance of the solenoid is (Neglect the field variation cross-section of the toroid)
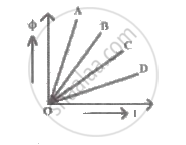
A graph of magnetic flux `phi` versus current (I) is shown for four inductors A, B, C, D. Smaller value of self inductance is for inductor ____________.
The self-inductance associated with a coil is independent of ______.
Energy needed to establish an alternating current I in a coil of self-inductance L is
In a fluorescent lamp choke (a small transformer) 100 V of reverse voltage is produced when the choke current changes uniformly from 0.25 A to 0 in a duration of 0.025 ms. The self-inductance of the choke (in mH) is estimated to be ______.
The inductance of a solenoid L having diameter d. Let n be the number of turns per unit length. The inductance per unit length near the middle of a solenoid is ______.
(Assume that, l = current passes through the turns, µ0 = Permeability of vacuum)
Calculate the self-inductance of a coil using the following data obtained when an AC source of frequency `(200/pi)` Hz and a DC source are applied across the coil.
| AC Source | ||
| S.No. | V (volts) | I (A) |
| 1 | 3.0 | 0.5 |
| 2 | 6.0 | 1.0 |
| 3 | 9.0 | 1.5 |
| DC Source | ||
| S.No. | V (volts) | I (A) |
| 1 | 4.0 | 1.0 |
| 2 | 6.0 | 1.5 |
| 3 | 8.0 | 2.0 |
Obtain an expression for the self inductance of a solenoid.
