Advertisements
Advertisements
प्रश्न
D and E are points on the sides AB and AC respectively of Δ ABC such that AB=5.6cm, AD= 1.4cm, AC=7 .2cm and AE = 1.5 cm, show that DE is parallel to BC
उत्तर
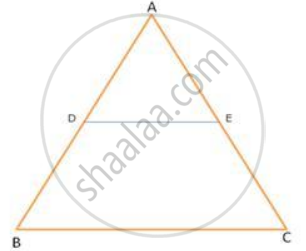
To prove : DE || BC
Sol : AB = 5.6 cm AC = 7.2 cm
AD = 1.4 cm AE = 1.8 cm
DB = 4.2 cm EC = 5.4 cm
`"AD"/"DB" = 1.4/4.2 = 1/3` ...(1)
`"AE"/"EC" = 1.8/5.4 = 1/3` ....(2)
From (1) and (2)
`"AD"/"DB" = "AE"/"EC"`
∴ DE || BC (by concerse of BPT)
APPEARS IN
संबंधित प्रश्न
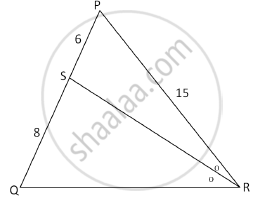
In the following figure, in ΔPQR, seg RS is the bisector of ∠PRQ. If PS = 6, SQ = 8, PR = 15, find QR.
The diagonals of a quadrilateral ABCD intersect each other at the point O such that `("AO")/("BO") = ("CO")/("DO")`. Show that ABCD is a trapezium.
ABCD is a quadrilateral in which AD = BC. If P, Q, R, S be the midpoints of AB, AC, CD and BD respectively, show that PQRS is a rhombus.

ΔABC~ΔPQR and ar(ΔABC) = 4, ar(ΔPQR) . If BC = 12cm, find QR.
In ΔABC, point D divides AB in the ratio 5:7, Find: DE, If BC = 4.8cm
In ΔABC, BP and CQ are altitudes from B and C on AC and AB respectively. BP and CQ intersect at O. Prove that
(i) PC x OQ = QB x OP
(ii) `"OC"^2/"OB"^2 = ("PC" xx "PO")/("QB" xx "QO")`
In any triangle _______ sides are opposite to equal angles
In fig., seg AC and seg BD intersect each other at point P.

`"AP"/"PC" = "BP"/"PD"` then prove that ΔABP ~ ΔCDP
∆ABC ~ ∆PQR. If AM and PN are altitudes of ΔABC and ∆PQR respectively and AB2 : PQ2 = 4 : 9, then AM : PN = ______.
In the adjoining diagram the length of PR is ______.

