Advertisements
Advertisements
प्रश्न
In MBC, DE is drawn parallel to BC. If AD: DB=2:3, DE =6cm and AE =3.6cm, find BC and AC.
उत्तर
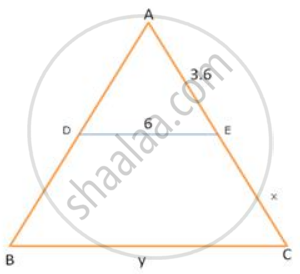
Given : DE = 6 cm , AE = 3.6 cm ,
To find : BC and AC
Sol : In Δ ABC , DE || BC
∴ By BPT
x =
= 1.8 × 3
x = 5.4 = EC
∴ AC = 3.6 + 5.4 = 9 cm
AC = 9 cm
In ΔADE and Δ ABC
∠ ADE = ∠ ABC
Similarly ∠AED = ∠ ACB (corresponding angles)
∴ ΔADE ∼ Δ ABC (AA corollary)
y =
y = 15
BC = 15 cm
APPEARS IN
संबंधित प्रश्न
In ∆ABC, ray BD bisects ∠ABC and ray CE bisects ∠ACB. If seg AB ≅ seg AC then prove that ED || BC.

O is any point in the interior of ΔABC. Bisectors of ∠AOB, ∠BOC and ∠AOC intersect side AB, side BC, side AC in
F, D and E respectively.
Prove that
BF × AE × CD = AF × CE × BD
Δ ABC - Δ XYZ. If area of Δ ABC is 9cm2 and area of Δ XYZ is 16cm2 and if BC= 2.1cm, find the length of YZ.
Figure shows Δ KLM , P an T on KL and KM respectively such that∠ KLM =∠ KTP.
If

In the following figure, M is mid-point of BC of a parallelogram ABCD. DM intersects the diagonal AC at P and AB produced at E. Prove that : PE = 2 PD

In a right-angled triangle ABC, ∠B = 90°, P and Q are the points on the sides AB and AC such as PQBC, AB = 8 cm, AQ = 6 cm and PA:AB = 1:3. Find the lengths of AC and BC.
Find the scale factor in each of the following and state the type of size transformation:
Image length = 6cm, Actual length = 4cm.
If BD ⊥ AC and CE ⊥ AB, prove that ∆AEC ~ ∆ADB

A flag pole 15 m high casts a shadow of 3 m at 10 a.m. The shadow cast by a building at the same time is 18.6 m. The height of the building is
In the given figure ΔABC ~ ΔPQR. The value of x is
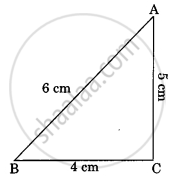 |
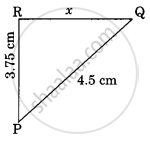 |
