Advertisements
Advertisements
प्रश्न
Derive the expression for the electric potential due to an electric dipole at a point on its axial line.
Advertisements
उत्तर
i) Let P be the point at which electric potential is required.
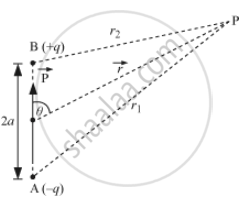
Potential at P due to − q charge,
`V_1=-q/(4piepsilon_0r_1)`
Potential at P due to + q charge,
`V_2=q/(4piepsilon_0r_2)`
Potential at P due to the dipole,
`V=V_1+V_2`
`V=q/(4piepsilon_0)[1/r_2-1/r_1]` .....(i)
Now, by geometry
`r_1^2=r^2+a^2+2arcostheta`
`r_2^2=r^2+a^2+2arcos(180^@-theta)`
`r_2^2=r^2+a^2-2arcostheta`
`r_1^2=r^2(1+a^2/r^2+(2a)/rcostheta)`
If r >> a, `a/r` is small. Therefore,`a^2/r^2` can be neglected.
`r_1^2=r^2(1+(2a)/rcostheta)`
`1/r_1=1/r(1+(2a)/rcostheta)^(1/2)`
similarly, `1/r_2=1/r(1-(2a)/rcostheta)^(1/2)`
Putting these values in (i), we obtain
`V=q/(4piepsilon_0)[1/r(1-(2a)/rcostheta)^(1/2)-1/r(1+(2a)/rcostheta)^(1/2)]`
Using Binomial theorem and retaining terms up to the first order in`a/r`, we obtain
`V=q/(4piepsilon_0r)[1+a/rcostheta-(1-a/rcostheta)]`
`V=q/(4piepsilon_0r)[1+a/rcostheta-1+a/rcostheta]`
`V=q/(4piepsilon_0r)[(2a)/rcostheta]`
`V=(qxx2acostheta)/(4piepsilon_0r^2)`
`V=(Pcostheta)/(4piepsilon_0r^2)`
For axial line put θ=0°
`V=(Pcos(0))/(4piepsilon_0r^2)`
`V=P/(4piepsilon_0r^2)`
APPEARS IN
संबंधित प्रश्न
A short electric dipole (which consists of two point charges, +q and -q) is placed at the centre 0 and inside a large cube (ABCDEFGH) of length L, as shown in Figure 1. The electric flux, emanating through the cube is:
a) `q"/"4piin_9L`
b) zero
c) `q"/"2piin_0L`
d) `q"/"3piin_0L`
- Define torque acting on a dipole of dipole moment \[\vec{p}\] placed in a uniform electric field \[\vec{E}\] Express it in the vector from and point out the direction along which it acts. Express it in the vector from and point out the direction along which it acts.
- What happens if the field is non-uniform?
- What would happen if the external field
\[\vec{E}\] is increasing (i) parallel to \[\vec{p}\] and (ii) anti-parallel to \[\vec{p}\]?
Two particles A and B, of opposite charges 2.0 × 10−6 C and −2.0 × 10−6 C, are placed at a separation of 1.0 cm. Two particles A and B, of opposite charges 2.0 × 10−6 C and −2.0 × 10−6 C, are placed at a separation of 1.0 cm.
Two particles A and B, of opposite charges 2.0 × 10−6 C and −2.0 × 10−6 C, are placed at a separation of 1.0 cm. Calculate the electric field at a point on the perpendicular bisector of the dipole and 1.0 m away from the centre.
A metal sphere of radius 1 cm is given a charge of 3.14 µC. Find the electric intensity at a distance of 1 m from the centre of sphere.
`[epsilon_0 = 8.85 xx 10^-12 "F"//m]`
Electric charges q, q, - 2q are placed at the comers of an equilateral triangle ABC of side l. The magnitude of electric dipole moment of the system is ____________.
The electric potential V as a function of distance X is shown in the figure.
The graph of the magnitude of electric field intensity E as a function of X is ______.
A square surface of side l (m) in the plane of the paper. A uniform electric field E(V/m) also in the plane of the paper is limited only to the lower half of the square surface, the electric flux (in SI units) associated with the surface is ______.
