Advertisements
Advertisements
प्रश्न
A semicircular wire has a length L and mass M. A particle of mass m is placed at the centre of the circle. Find the gravitational attraction on the particle due to the wire.
Advertisements
उत्तर
Consider a small mass element of length dl subtending dθ angle at the centre.
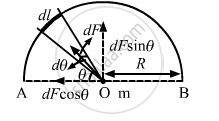
In the semicircle, we can consider a small element dθ.
Then length of the element, dl = R dθ
Mass of the element, dm \[= \left( \frac{M}{L} \right)R d\theta\]
Force on the mass element is given by
\[dF = \frac{Gm}{R^2}dm = \frac{GMRm}{L R^2}{d}{\theta}\]
The symmetric components along AB cancel each other.
Now, net gravitational force on the particle at O is given by
\[F = \int2dF\sin \theta\]
\[ = \int\frac{2GMm}{LR}\sin \theta d\theta\]
\[ \therefore F = \int_0^\pi/2 \frac{- 2GMm}{LR}\sin \theta d\theta\]
\[ = \frac{2GMm}{LR} \left[ - \cos \theta \right]_0^\pi/2 \]
\[ = - 2\frac{GMm}{LR}\left( - 1 \right)\]
\[ = \frac{2GMm}{LR} = \frac{2GMm}{LL/\pi}\]
\[ = \frac{2\pi G Mm}{L^2}\]
APPEARS IN
संबंधित प्रश्न
Choose the correct alternative:
Acceleration due to gravity increases/decreases with increasing depth. (assume the earth to be a sphere of uniform density).
Choose the correct alternative:
Acceleration due to gravity is independent of mass of the earth/mass of the body.
State and explain Kepler's laws of planetary motion. Draw diagrams to illustrate these laws.
At noon, the sun and the earth pull the objects on the earth's surface in opposite directions. At midnight, the sun and the earth pull these objects in same direction. Is the weight of an object, as measured by a spring balance on the earth's surface, more at midnight as compared to its weight at noon?
A body is suspended from a spring balance kept in a satellite. The reading of the balance is W1 when the satellite goes in an orbit of radius R and is W2 when it goes in an orbit of radius 2 −R.
A thin spherical shell having uniform density is cut in two parts by a plane and kept separated as shown in the following figure. The point A is the centre of the plane section of the first part and B is the centre of the plane section of the second part. Show that the gravitational field at A due to the first part is equal in magnitude to the gravitational field at B due to the second part.
The gravitational field in a region is given by \[E = \left( 2 \overrightarrow{i} + 3 \overrightarrow{j} \right) N {kg}^{- 1}\] . Show that no work is done by the gravitational field when a particle is moved on the line 3y + 2x = 5.
[Hint : If a line y = mx + c makes angle θ with the X-axis, m = tan θ.]
A ball is thrown vertically upwards. It goes to a height 20 m and then returns to the ground. Taking acceleration due to gravity g to be 10 ms-2 , find :
the total time of journey of the ball .
How will the force of gravitation between two objects change if the distance between them is:
Doubled
How will the force of gravitation between two objects change if the distance between them is:
Made four times
Why don't you feel the force of attraction between your friend sitting close to you and yourself?
A ball is thrown up with a speed of 4.9 ms-1.
Prove that the time of ascent is equal to the time of descent.
A force can produce ________, In an object at rest. It can __________ an object and change its __________ of motion.
Gravity is another kind of ________. It exerts all through the ________. The Sun's gravity keeps the ___________ in their orbits. Gravity can only be felt with very large ________.
An apple falls towards the earth due to its gravitational force. The apple also attracts the earth with the same force. Why do we not see the earth rising towards the apple? Explain.
The value of universal gravitational constant (G) in the SI unit is ______.
Give the applications of universal law gravitation.
Particles of masses 2M, m and M are respectively at points A, B and C with AB = ½ (BC). m is much-much smaller than M and at time t = 0, they are all at rest (Figure). At subsequent times before any collision takes place ______.
If the law of gravitation, instead of being inverse-square law, becomes an inverse-cube law- ______.
- planets will not have elliptic orbits.
- circular orbits of planets is not possible.
- projectile motion of a stone thrown by hand on the surface of the earth will be approximately parabolic.
- there will be no gravitational force inside a spherical shell of uniform density.
Ocean tides on Earth are mainly caused by ______.
