Advertisements
Advertisements
प्रश्न
Draw a schematic sketch of an ac generator describing its basic elements. State briefly its working principle. Show a plot of variation of
(i) Magnetic flux and
(ii) Alternating emf versus time generated by a loop of wire rotating in a magnetic field.
उत्तर
Principle − Based on the phenomenon of electromagnetic induction.
Construction:

Main parts of an ac generator:
Armature − The rectangular coil ABCD
Filed Magnets − Two pole pieces of a strong electromagnet
Slip Rings − The ends of the coil ABCD are connected to two hollow metallic rings R1 and R2.
Brushes − B1 and B2 are two flexible metal plates or carbon rods. They are fixed and are kept in tight contact with R1 and R2, respectively.
Working − As the armature coil is rotated in the magnetic field, angle θ between the field and the normal to the coil changes continuously. Therefore, magnetic flux linked with the coil changes and an emf is induced in the coil. According to Fleming’s right hand rule, current is induced from A to B in AB and from C to D in CD. In the external circuit, current flows from B2 to B1.
To calculate the magnitude of emf induced:
Suppose A → Area of each turn of the coil
N → Number of turns in the coil
`vecB`→ Strength of the magnetic field
θ → Angle which normal to the coil makes with `vecB` at any instant t
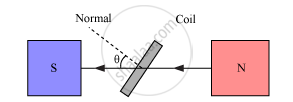
∴ Magnetic flux linked with the coil in this position is given by,
`phi=N(vecB.vecA)=NBAcostheta=NBAcos `
Where, ‘ω’ is angular velocity of the coil
Graph between magnetic flux and time, according to equation (i), is shown below:

As the coil rotates, angle θ changes. Therefore, magnetic flux Φ linked with the coil changes and an emf is induced in the coil. At this instant t, if e is the emf induced in the coil, then
`e=(dtheta)/dt=-d/dt(NABcos`
`=-NABd/dt(cos `
=-NAB(-sin ωt)ω
∴ e = NAB ω sin ωt
The graph between alternating emf versus time is shown below:

APPEARS IN
संबंधित प्रश्न
A square loop MNOP of side 20 cm is placed horizontally in a uniform magnetic field acting vertically downwards as shown in the figure. The loop is pulled with a constant velocity of 20 cm s−1 till it goes out of the field.
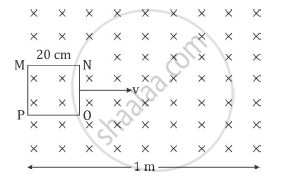
(i) Depict the direction of the induced current in the loop as it goes out of the field. For how long would the current in the loop persist?
(ii) Plot a graph showing the variation of magnetic flux and induced emf as a function of time.
Calculate magnetic flux density of the magnetic field at the centre of a circular coil of 50 turns, having a radius of 0.5m and carrying a current of 5 A.
The magnetic flux linked with the coil (in Weber) is given by the equation- Փ = 5t2 + 3t + 16. The induced EMF in the coil at time, t = 4 will be ______.
The magnetic flux linked with a coil in Wb is given by the equation Φ = 3t2 + 4t + 9. Then the magnitude of induced emf at t = 2 sec will be ______.
The dimensions of magnetic flux are ______
The unit of magnetic flux in SI is ______
A square of side L meters lies in the x-y plane in a region, where the magnetic field is given by `B = Bo(2hati + 3hatj + 4hatk)`T, where B0 is constant. The magnitude of flux passing through the square is ______.
A coil is placed in a time varying magnetic field. If the number of turns in the coil were to be halved and the radius of wire doubled, the electrical power dissipated due to the current induced in the coil would be: (Assume the coil to be short circuited.)
