Advertisements
Advertisements
प्रश्न
Draw the graph of the following function:
f(x) = `|x|/x`
उत्तर
If f: R → R is defined by
f(x) = `{(|x|/x if x ≠ 0),(0 if x = 0):}`
= `{(x/x if x > 0),((- x)/x if x < 0):}`
= `{(1 if x > 0),(- 1 if x < 0):}`
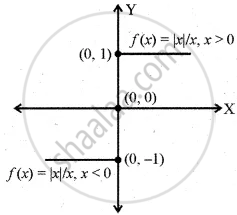
The domain of the function is R and the range is {-1, 0, 1}.
APPEARS IN
संबंधित प्रश्न
Determine whether the following function is odd or even?
f(x) = sin x + cos x
Determine whether the following function is odd or even?
f(x) = x2 – |x|
If f(x) = `x^3 - 1/x^3`, then show that `"f"(x) + "f"(1/x)` = 0
Draw the graph of the following function:
f(x) = 16 – x2
If f(x) = `1/(2x + 1)`, x > `-1/2`, then show that f(f(x)) = `(2x + 1)/(2x + 3)`
Draw the graph of y = 9 - x2.
If f(x) = x2 – x + 1 then f(x + 1) is:
The graph of y = ex intersect the y-axis at:
The range of f(x) = |x|, for all x ∈ R is:
The graph of f(x) = ex is identical to that of:
