Advertisements
Advertisements
प्रश्न
Factorise the following expressions
m2 + m – 72
उत्तर
m2 + m – 72
This is of the form ax + bx + c
where a = 1, b = 1, c = – 72
| Product = – 72 | Sum = 1 |
| 1 × (– 72) = – 72 | 1 + (– 72) = – 71 |
| 2 × (– 36) = – 72 | 2 + (– 36) = – 34 |
| 3 × (– 24) = – 72 | 3 + (– 24) = – 21 |
| 4 × (– 18) = – 72 | 4 + (– 18) = – 14 |
| 6 × (– 12) = – 72 | 6 + (– 12) = – 6 |
| 8 × (– 9) = – 72 | 8 + (– 9) = – 1 |
| 9 × (– 8) = – 72 | 9 + (– 8) = 1 |
Product a × c = 1 × – 72 = – 72
Sum b = 1
The middle term m can be written as 9m – 8m
m2 + m – 72 = m2 + 9m – 8m – 72
= m(m + 9) – 8(m + 9)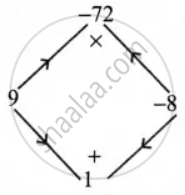
Taking out (m + 9)
= (m + 9)(m – 8)
∴ m2 + m – 72 = (m + 9)(m – 8)
APPEARS IN
संबंधित प्रश्न
Expand: 102 x 98
(a + b)(a – b) = a2 – b2
Factorise the following using the identity a2 – b2 = (a + b)(a – b).
28ay2 – 175ax2
Factorise the following using the identity a2 – b2 = (a + b)(a – b).
49x2 – 36y2
Factorise the following using the identity a2 – b2 = (a + b)(a – b).
x4 – y4
Factorise the following using the identity a2 – b2 = (a + b)(a – b).
x4 – y4 + x2 – y2
Factorise the following using the identity a2 – b2 = (a + b)(a – b).
9x2 – (3y + z)2
Verify the following:
(a + b + c)(a2 + b2 + c2 – ab – bc – ca) = a3 + b3 + c3 – 3abc
Find the value of `(198 xx 198 - 102 xx 102)/96`
The product of two expressions is x5 + x3 + x. If one of them is x2 + x + 1, find the other.
